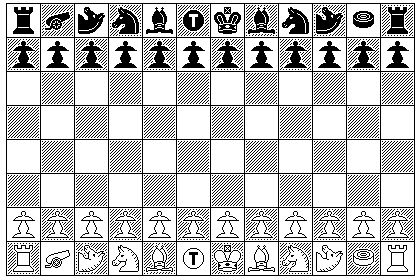
Here's another alternate form of Chess, only slightly expanded, on a 12 by 8 board, and yet I think of some interest:

This variant is intended to occupy a place halfway between the old form of Chess, in which the Queen had not replaced the Fers, and the Bishop had not replaced the Dabbaba, and present-day Chess.
The pieces along the back rank are:
Rook, Cannon, Camel, Knight, Bishop, Tiger, King, Bishop, Knight, Camel, Cavalier, Rook
The powerful Queen is replaced by the less powerful Tiger, which moves, but does not capture, like a Bishop, and which captures, but does not move without capturing, as a Knight.
The Camel is, as we've seen, a piece that moves like the Knight, but the long part of its move is one square longer, resulting in a displacement of one square in one orthogonal direction, and three squares in a perpendicular orthogonal direction.
The Cavalier, depicted in the diagram by a checker, moves one square orthogonally or diagonally, but does not capture in this fashion. It may jump over an allied man to move two squares without disturbing that piece. As well, it may jump over an enemy piece, capturing it. It may also, in one move, make multiple jumps, all of the same kind (that is, all jumps over allied pieces, or all captures of enemy pieces), of either of these two kinds. As well, it may make one or more jumps over allied pieces strictly followed by one or more jumps over enemy pieces. (This move may be familiar as the "Knight's Charge" from the games of Chivalry and Camelot by Parker Brothers.) In either of these cases, however, either all of the jumps made during a single move must be diagonal, or all those jumps must be orthogonal. This is the one difference between the movement of this piece and that of the Checker in the first variant on this page.
The Cannon in this game is actually a Vao. It moves like a Bishop, and captures the way the Cannon does in Chinese Chess, by jumping over one intervening piece (the "screen"), but again along the diagonal lines along which a Bishop moves, rather than the orthogonal lines along which a Rook moves.
The Rook also has a changed move: it still moves orthogonally, but it can only make moves from one to five squares in length.
Castling is as in regular Chess; the King moves two squares, and the Rook, provided no pieces are intervening, moves to the space the King passed over.
The Pawn may move two spaces forward on its first move, and, as well, en passant capture is the same as in regular Chess.
As well, the places of the Cannon and the Cavalier may be interchanged once, immediately before the first move of either of those pieces (which means the other of which must also have not been moved). The purpose of this special move is because the King's side Cavalier can move without disturbing the wall of Pawns in front of what could become a Kingside castled position; thus, it facilitates Queenside - or, rather, Tiger side - castling.
Thus, a single move may, when neither a player's Cannon nor the same player's Cavalier, has been moved, consist of first interchanging that Cannon and Cavalier, and then moving one of them for the first time.
Also, if the Cannon and Cavalier are interchanged before the first move of either, that first move may not be a capture. One consequence of this restriction is that the Cannon only pins the opposing player's King's Bishop Pawn at the start of the game, and not the Tiger's Bishop Pawn as well.
Thus, as the Queen has been replaced by a piece with roughly the power of a minor piece, and the Rook has been limited in range, it is presumed to be less likely that a game will be decided in the first few moves, thus, it is hoped, reducing the tendency to stereotyped openings.
The Tiger changes color with every capture, and the Cannon and even the Cavalier have sufficiently unusual moves to add interest and surprises to the game.
However, it may be felt that having only one piece, the Cavalier, with a move like a checker instead of a chess piece is a defect; it could be replaced with a piece that moves as a Knight and captures as a Bishop, the inverse of the Tiger - or by what may be a somewhat weaker piece, one that moves as a Knight and captures as a King. Either way, moving as a Knight means that the same property with respect to Castling is retained.

Or the whole problem can be avoided by an even simpler expedient: have two Cannons (diagonal Cannons, each one really a Vao), instead of a Cannon and a Cavalier... but allow each Cannon to make a Knight's move as its first move. That, of course, changes its color, so allowing both of them to do this allows the player to retain two Cannons of opposite color.
And let's add one more special rule to the game.
To make the game more interesting, by eliminating the advantage that White has from the first move, an obvious possibility would be to only allow White to make half a move for the first move. This, of course, isn't really possible, but an obvious close approach would be to deny White the privilege of making a two-step Pawn move for the first move.
This, however, would be likely to have the effect of reducing the diversity of openings; White would end up always making a Knight move as his first move.
So, for regular Chess, a somewhat more complicated scheme might work out better.
If White's first move involves advancing one of the center four Pawns, that Pawn may not be advanced two steps. If White's first move involves advancing one of the other Pawns, it may be advanced by one or two spaces, as is normal. If White's first move is a Knight move, then whenever White does make his first Pawn move (of any Pawn, even an edge Pawn), that Pawn may only be advanced by one step. Thus, choosing to advance one of the edge Pawns, generally considered a weaker move, is rewarded with the handicap for White being removed; but opening with a Knight move instead only delays the loss of half a move.
For this version of chess, instead of the central four Pawns starting out with the restriction, it would be the central six Pawns.
Also, stalemate can be scored as 3/5 of a win; with the other step to eliminate White's advantage, Dynamic Scoring would not be required.