On previous pages, we have encountered some of the elements of the calendar system of the Maya, elements of which were shared with other civilizations in Mesoamerica.
One element of the Maya calendar was the Tzolkin, a series of 260 days which resulted from the interplay between two kinds of week, a week of 13 days and a week of 20 days. Perpetual calendars for these two weeks were given on this page. It was called the tonalpohualli by the Aztecs, who continued to use it.
The days of the week of 13 days were simply numbered from 1 through 13.
The days of the week of 20 days were named:
Mayan Aztec
1) Imix Cipactli Crocodile
2) Ix Ehecatl Wind
3) Akbal Calli House
4) Kan Cuetzpalin Lizard
5) Chicchan Coatl Snake
6) Cimi Miquiztli Death
7) Manik Mazatl Deer
8) Lamat Tochtli Rabbit
9) Muluc Atl Water
10) Oc Itzcuintli Dog
11) Chuen Ozomahtli Monkey
12) Eb Malinalli Grass
13) Ben Acatl Reed
14) Ix Ocelotl Jaguar
15) Men Cuauhtli Eagle
16) Cib Cozcacuauhtli Vulture
17) Caban Ollin Quake
18) Etznab Tecpatl Flint
19) Cauac Quiahuitl Rain
20) Ahau Xochitl Flower
The English versions of the Aztec names are a common conventional set, not in all cases an accurate translation.
Another element was the Haab. This was a year of 365 days without any leap years; thus, it was discussed, along with the ancient Egyptian vague year, in the page concerning Julian Day numbers. The Aztecs used it, referring to it as the xiuhpohualli.
This year consisted of eighteen months, each 20 days long, plus one epagomenal period of five days which went by the name Uayeb.
Its months were named:
Mayan Aztec
1) Pop Chief Atlcahualo Ceasing Water
2) Uo Night Jaguar Tlacaxipehualiztli Fertility
3) Zip Cloud Serpent Tozoztontli Small
4) Zotz Bat Hueytozoztli Great
5) Tzec Sky and Earth Toxcatl Dryness
6) Xul Dog Etzalcualiztli Corn and Beans
7) Yaxkin First Sun Tecuilhuitontli Revered Ones
8) Mol Collection Hueytecuilhuitl Greatly Revered Ones
9) Chen Cave of the Moon Tlaxochimaco Revered Deceased
10) Yax New Xocotlhuetzin Greatly Revered Deceased
11) Sac Frog Ochpaniztli Sweeping
12) Ceh Red Deer Teoleco Return of the Gods
13) Mac Enclosure Tepeihuitl Mountains
14) Kankin Dog of the Underworld Quecholli Precious Feather
15) Muan Bird Panquetzalitli Raising the Banners
16) Pax Great Puma Atemotzli Descent of the Water
17) Kayab Turtle Tititl Stretching
18) Cumhu Underworld Dragon Izcalli Encouragement
Uayeb Nemontemi
The Tzolkin, and possibly also the Haab, date back at least to the Olmecs, a civilization which preceded the Maya, and may also have been used by the Zapotec.
Note that which month of the Aztec year was the first month is a matter of some controversy. Atlcahualo is shown here as the first month, as was set forth by Bernardino de Sahagún; Antonio de León y Gama had Tititl as the first month, and Mariano Fernández Echeverría y Veytia had Atemotzli.
There is a third component to the Mayan calendar, the Long Count.
In this system, we have:
Julian Julian Day Long Count Tzolkin Haab September 5, 3114 B.C. 584283 19.19.19.17.19 3 Cauac 7 Cumhu September 6, 3114 B.C. 584284 0. 0. 0. 0. 0 4 Ahau 8 Cumhu
according to the generally-accepted correlation between the Mayan calendar and Western dates. As the Long Count is a strict count of days, its first three numbers give the number of a 360-day year. However, 8,000 360-day years do not add up to an even number of 365-day years or 260-day years, and the Long Count is, at least according to one source I have encountered, to always begin on 4 Ahau 8 Cumhu.
Note that the date September 6, 3114 B.C. began at midnight of the previous day, J.D. 584283.5; at noon on that date, it was J.D. 584284, and at midnight, J.D. 584284.5. Thus, in my discussion of the Julian Day, I have treated that day as J.D. 584284; other sources will represent a calendar day by truncating the Julian Day number at the start of the day, referring to it as J.D. 584283. Thus, while I represent the day 0.0.0.0.0 as Julian Day 584284, I am correlating it to the day September 6, 3114 B.C. in the Julian calendar, and therefore I am using what is sometimes called the 584283 correlation.
It may also be noted that the year 2012 is not 8,000 years, even short years of 360 days, from 3113 B.C.; thus, the Mayan belief that the world is destroyed and created anew with the starting of each new calendar round would apply to Julian Day 3,464,649, or October 14, 4773 AD, Gregorian, if the Goodman-Martinez-Thompson correlation is correct.
I have chosen the form of the GMT correlation that begins the Long Count on September 6, 3114 B.C., as originally proposed by Goodman; Martinez proposed it to start one day later, and Thompson two days later. The original Goodman correlation has the advantage that it leads to the Tzolkin, as used by the Classical Maya, remaining synchronized to the Tzolkin as used by Maya communities today.
While one source had claimed that the Tzolkin of the GMT correlation matches that used by Maya communities today, other information shows the Quiché Maya of today using a Tzolkin that is one day ahead of the GMT (thus matching the M in GMT), and the Yucatec Maya of today using a Tzolkin that is fifty days ahead of the GMT.
The Quiché Maya of today have been claimed by one source to adjust their Haab to the Gregorian calendar, but another source has them maintaining the ancient Haab tradition, but with the change of placing the five epagomenal days of Uayeb between Pax and Kayab.
The Spinden correlation assigns the origin of the Mayan calendar to a date five 52-year calendar rounds earlier than September 7, 3114 B.C., and the Vaillant correlation assigns it to a date five 52-year calendar rounds later than September 6, 3114 B.C., so the Vaillant correlation and a Spinden - 1 correlation would agree with the Goodman correlation in both Haab and Tzolkin. Also, the Bowditch correlation matches the Tzolkin but not the Haab, and the Kreichgauer correlation is only a few days away from the Tzolkin as currently in use as well.
However, I had overlooked one thing, and it turns out the people claiming that the end of the world is coming in the year 2012 aren't depending on the world coming to an end every 52 years after all. The world, after all, didn't come to an end in 1960; it didn't even come to an end in, say, 1964.
Since the long count always begins on 4 Ahau 8 Cumhu, it follows that it needs to be a multiple of 52 years. 8,000 years isn't a multiple of 52, but 13 times 400 would be a multiple of 52. 5,200 short years of 360 days is 1,872,000 days; but it works out to 5,128 vague years of 365 days with 280 days left over. So having the long count end on 12.19.19.17.19 and begin over at 0.0.0.0.0 doesn't quite work out either.
Actually, this is an invalid objection to the December 21, 2012 date. What is actually going on should be obvious. Currently, each calendar round is held to start on 4 Ahau 8 Cumhu because we are in a Long Count era that started on 4 Ahau 8 Cumhu. Since December 21, 2012, the beginning of the new Long Count era, falls on 4 Ahau 3 Yax, henceforth, in the new age that it will herald, the date on which the beginning of a new calendar round will be celebrated will be 4 Ahau 3 Yax. |
On further reflection, it is even more complicated than that. After all, the beginning of each Calendar Round is celebrated on 4 Ahau 8 Cumhu, the last 0.0.0.0.0 date, not 11 Ahau 13 Ceh. Thus, while December 21, 2012 was to have indicated the destructive end of an era after thirteen baktuns within the current pictun, presumably what was to follow would be seven baktuns of darkness and savagery before we climed back up to civilization, and resumed using the Mayan calendar, starting the Long Count from October 14, 4772 A.D., which falls on 10 Ahau 13 Yaxkin, which is when each Calendar Round would begin in the new pictun. |
Of course, if the five unlucky epagomenal days of Uayeb were not counted in the long count we could then wait 5,200 vague years of 365 days, and there would be no problem. However, a large number of monuments left by the Maya which include dates in the Long Count form accompanied by the corresponding Haab and Tzolkin eliminate that possibility quite conclusively.
On top of everything else, there are 20 baktun in a piktun, not 13. However, that the date 13.0.0.0.0 was believed to be the end of an age by the Maya apparently is, to my surprise, attested to some extent by reputable sources, such as the book Skywatchers, by Anthony Aveni. Apparently it is unclear if the baktun advances from 19 to 0 or from 13 to 1 from extant inscriptions. But that a start date of 4 Ahau 8 Cumhu is required for a new age is also affirmed by the sources I have recently been consulting. (I was hoping to debunk the 2012 panic thoroughly by showing it to be wholly a New Age invention without any genuine Mayan roots.) The Aztecs, on the other hand, did believe that every time the 52-year Calendar Round came to an end, there was a risk of the world coming to an end, which had to be averted by ceremony and ritual.
If one waits long enough, one can find a date on which the world can come to an end:
Julian Julian Day Long Count Tzolkin Haab
December 4, 5874 B.C, -423,717 0. 1.12.19.19.19.19.17.19 10 Cauac 12 Ceh
December 5, 5874 B.C, -423,716 0. 1.12.19.13. 0. 0. 0. 0 11 Ahau 13 Ceh
September 5, 3114 B.C. 584,283 0. 1.12.19.19.19.19.17.19 3 Cauac 7 Cumhu
September 6, 3114 B.C. 584,284 0. 1.13. 0. 0. 0. 0. 0. 0 4 Ahau 8 Cumhu
July 19, 690 A.D. 1,973,280 0. 1.13. 0. 9.12.18. 5.16 2 Cib 14 Mol
July 21, 690 A.D. 1,973,282 0. 1.13. 0. 9.12.18. 5.18 4 Etznab 16 Mol
Gregorian
March 24, 1980 A.D. 2,444,323 0. 1.13. 0.12.18. 6.13.19 3 Cauac 7 Cumhu
March 25, 1980 A.D. 2,444,324 0. 1.13. 0.12.18. 6.14. 0 4 Ahau 8 Cumhu
December 21, 2012 A.D. 2,456,283 0. 1.13. 0.12.19.19.17.19 3 Cauac 2 Yax
December 22, 2012 A.D. 2,456,284 0. 1.13. 0.13. 0. 0. 0. 0 4 Ahau 3 Yax
March 11, 2032 A.D. 2,463,303 0. 1.13. 0.13. 0.19. 8.19 3 Cauac 7 Cumhu
March 12, 2032 A.D. 2,463,304 0. 1.13. 0.13. 0.19. 9. 0 4 Ahau 8 Cumhu
February 27, 2084 A.D. 2,482,283 0. 1.13. 0.13. 3.12. 3.19 3 Cauac 7 Cumhu
February 28, 2084 A.D. 2,482,284 0. 1.13. 0.13. 3.12. 4. 0 4 Ahau 8 Cumhu
October 19, 2272 A.D. 2,551,183 0. 1.13. 0.13.13. 3.10.19 3 Cauac 2 Yax
October 20, 2272 A.D. 2,551,184 0. 1.13. 0.13.13. 3.11. 0 4 Ahau 3 Yax
October 13, 4772 A.D. 3,464,283 0. 1.13. 0.19.19.19.17.19 9 Cauac 12 Yaxkin
October 14, 4772 A.D. 3,464,284 0. 1.13. 1. 0. 0. 0. 0. 0 10 Ahau 13 Yaxkin
March 8, 15,595 A.D. 7,417,083 0. 1.13. 2. 7. 8.19.17.19 3 Cauac 7 Cumhu
March 9, 15,595 A.D. 7,417,084 0. 1.13. 2. 7. 9. 0. 0. 0 4 Ahau 8 Cumhu
January 12, 371,039 A.D. 137,240,283 0. 1.15. 7. 8.19.19.17.19 3 Cauac 7 Cumhu
January 13, 371,039 A.D. 137,240,284 0. 1.15. 7. 9. 0. 0. 0. 0 4 Ahau 8 Cumhu
January 5, 7,479,916 A.D. 3,733,704,283 0. 4. 2. 8 19.19.19.17.19 3 Cauac 7 Cumhu
January 6, 7,479,916 A.D. 3,733,704,284 0. 4. 2. 9. 0. 0. 0. 0. 0 4 Ahau 8 Cumhu
| | | | | | | | |
| | | | | | | | --- kin (day)
| | | | | | | ------ uinal (20-day month)
| | | | | | --------- tun (360-day short year)
| | | | | ------------ katun (20 years)
| | | | --------------- baktun (400 years)
| | | ------------------ pictun (8,000 years)
| | --------------------- calabtun (160,000 years)
| ------------------------ kinchiltun (3,200,000 years)
--------------------------- alautun (64,000,000 years)
The fact that the Mayans used a 20-day month is surprising, as it makes it appear that they ignored the phases of the moon in their calendar. This is not the case, however.
July 19, 690 A.D. is noted above as it is the date of a conjunction involving Mars, Jupiter, and Saturn that was recorded by the Maya.
Since a Pictun is 20 Baktuns, and not 13 Baktuns, perhaps the previous end of the world took place on December 4, 5874 B.C., rather than on September 5, 3114 B.C.; that would place it before what was at least once thought of the start of the civilizations of Egypt and Babylon, making the notion of the end of the world slightly more plausible... until, of course, December 21, 2012 has come and gone.
Incidentally, it might be noted that the first Egyptian dynastic pharaoh, Menes, is sometimes thought to have begun his reign on about 3100 B.C. (at least if he was the first one, Narmer, and not the second one, Hor-Aha), adding to the plausibility of that as a date for the re-emergence of civilization, seven baktuns after its destruction.
But then there's always the Vaillant correlation, noted above, which would delay the end of the world by 260 Haabs, until October 19, 2272 A.D..
The Aztec calendar stone records the previous occasions on which the world ended. Thus, while the significance of December 21, 2012 to the Maya might simply be similar to that of a new millenium, as some have claimed, and not actually imply any type of "end of the world" scenario, the Aztecs very definitely did think in terms of their calendar predicting possible destructive events. One recorded legend of these events divides history as follows:
Age Calendar Name Name Duration Starting
years rounds Year
First Sun 4 Jaguar 4 Ocelotl Tezcatlipoca Smoking Mirror 676 13 956 BC
Second Sun 4 Wind 4 Ehecatl Quetzalcoatl Feathered Serpent 364 7 280 BC
Third Sun 4 Rain 4 Quiahuitl Tlaloc 312 6 85 AD
Fourth Sun 4 Water 4 Atl Chalchiuhtlicue Lady of the Jade Skirts 676 13 397 AD
Fifth Sun 4 Quake 4 Ollin Tonatuih (current) 1073 AD
The First Sun was said to have begun 2513 years ago, when the story was recounted on May 22, 1558, which is what leads to the starting date of 956 BC shown in the table.
Thus, while the world doesn't normally end every 52 years, it does seem to come to an end more often according to the Aztecs than according to the Maya.
Apparently, the Aztec diligence in making human sacrifices averted the end of the world in the year 1749, but it must be considered overdue: 676 years later, the year 2425 is presumably a particularly dangerous one.
Perhaps the world did end in 1749, even if no one noticed, and it will also end during either 2061 or 2113 as well as by 2425, thus maintaining a more uniform frequency of apocalypses. (In 2061, 3 Etznab - the relevance of which will be explained below, basically it's the Mayan date on which the Aztec date of 4 Ollin falls - takes place on June 26. Of course, if the world came to an end on a date of 4 Ollin around 1749, we would be on the Sixth Sun now, and so another date would apply. Not having a more authentic source to turn to, it may be noted that if the author Thomas Harlan is to be believed, the Sixth Sun would come to an end on a 4 Flint or 4 Tecpatl date; that would be the equivalent of 3 Cauac, and that takes place in 2061 on May 18th.)
There is an ambiguity in the source (the "Leyenda de los Soles"), however, that might break up the division into 676 year periods. It may be that the flood which ended the period of the Fourth Sun was itself an additional 52-year interregnum between the end of the Fourth Sun and the beginning of the Fifth Sun.
As well, there are glyphs on the Aztec calendar stone that can be interpreted as giving the lengths of the Four Suns as 682, 530, 576, and 528 years respectively instead. That is, if those glyphs mean 52*13+6, 52*10+10, 52*11+4, and 52*10+8 respectively; they might also mean 400*13+6, 400*10+10, 400*11+4, and 400*10+8 instead, which would be 5206, 4010, 4404, and 4008 years.
Also, while the Aztec calendar, with the Xiuhpohualli corresponding to the Haab, and the Tonalpohualli corresponding to the Tzolkin, was based on the same principles as the Mayan calendar, it was not maintained in synchrony with it.
Correlations of the Aztec calendar with our own are generally based on the date given for the entry of Cortéz into Tenochtitlán, which took place on November 8, 1519 by the Julian calendar. This is definitely identified as taking place during the year 1 Acatl. As for the date, it was identified as 13 Quecholli; however, there is reason to believe that was the date by the calendar in Tescuco rather than the one in Tenochtitlán. The currently best accepted correlation takes 9 Quecholli as the date (this appears to derive from the work of Mariano Fernández Echeverría y Veytia); an older one, due to Antonio de León y Gama, gave 16 Quecholli.
The calendar for the year 1 Acatl would look like this:
1 2 3 4 5 6 7 8 9 10 11 12 13
Atlcahualo 1 2 3 4 5 6 7 8 9 10 11 12 13
14 15 16 17 18 19 20
Tlacaxipehualiztli 1 2 3 4 5 6
7 8 9 10 11 12 13 14 15 16 17 18 19
20
Tozoztontli 1 2 3 4 5 6 7 8 9 10 11 12
13 14 15 16 17 18 19 20
Hueytozoztli 1 2 3 4 5
6 7 8 9 10 11 12 13 14 15 16 17 18
19 20
Toxcatl 1 2 3 4 5 6 7 8 9 10 11
12 13 14 15 16 17 18 19 20
Etzalcualiztli 1 2 3 4
5 6 7 8 9 10 11 12 13 14 15 16 17
18 19 20
Tecuilhuitontli 1 2 3 4 5 6 7 8 9 10
11 12 13 14 15 16 17 18 19 20
Hueytecuilhuitl 1 2 3
4 5 6 7 8 9 10 11 12 13 14 15 16
17 18 19 20
Tlaxochimaco 1 2 3 4 5 6 7 8 9
10 11 12 13 14 15 16 17 18 19 20
Xocotlhuetzin 1 2
3 4 5 6 7 8 9 10 11 12 13 14 15
16 17 18 19 20
Ochpaniztli 1 2 3 4 5 6 7 8
9 10 11 12 13 14 15 16 17 18 19 20
Teoleco 1
2 3 4 5 6 7 8 9 10 11 12 13 14
15 16 17 18 19 20
Tepeihuitl 1 2 3 4 5 6 7
8 9 10 11 12 13 14 15 16 17 18 19 20
Quecholli 1 2 3 4 5 6 7 8 9 10 11 12 13
14 15 16 17 18 19 20
Panquetzalitli 1 2 3 4 5 6
7 8 9 10 11 12 13 14 15 16 17 18 19
20
Atemotzli 1 2 3 4 5 6 7 8 9 10 11 12
13 14 15 16 17 18 19 20
Tititl 1 2 3 4 5
6 7 8 9 10 11 12 13 14 15 16 17 18
19 20
Izcalli 1 2 3 4 5 6 7 8 9 10 11
12 13 14 15 16 17 18 19 20
Nemontemi 1 2 3 4
5
for the 13 day part of the 260 day cycle. As the named part involves a cycle of 20 days, and there are 20 days in the month, the correspondence will be the same for every month of a given year, and is shown below for the year 1 Acatl:
Acatl Reed 1 Ocelotl Jaguar 2 Cuauhtli Eagle 3 Cozcacuauhtli Vulture 4 Ollin Quake 5 Tecpatl Flint 6 Quiahuitl Rain 7 Xochitl Flower 8 Cipactli Crocodile 9 Ehecatl Wind 10 Calli House 11 Cuetzpalin Lizard 12 Coatl Snake 13 Miquiztli Death 14 Mazatl Deer 15 Tochtli Rabbit 16 Atl Water 17 Itzcuintli Dog 18 Ozomahtli Monkey 19 Malinalli Grass 20
Thus, 9 Quecholli of that year would be 9 Cipactli 9 Quecholli, and 16 Quecholli of that year would be 3 Tochtli 16 Quecholli.
November 8, 1519, by the Julian calendar, is, in the Mayan calendar, the date 10 Ahau 2 Pop, whereas 9 Cipactli 9 Quecholli is equivalent to 9 Imix 9 Kankin.
Knowing the offset between the Aztec calendar and the Maya calendar, which has the same structure, allows the existing tables for the Maya calendar to be used to look for Aztec dates.
To go from 10 Ahau to 9 Imix, the individual components change by -1 (or +12) and +1. Twelve days after 10 Ahau is 9 Eb; continuing to go by steps of 13 days, we get 9 Chicchan after twenty-five days, and 9 Etznab after thirty-eight days... reaching 9 Imix after one hundred and eighty-one days. Thus, the Tonalpohualli starts on day 182 of the Tzolkin, or the Tzolkin starts on day 80 of the Tonalpohualli.
9 Kankin is 267 days later in the Haab than 2 Pop, so the Xiuhpohualli starts on day 98 of the Haab, or the Haab starts on day 267 of the Xiuhpohualli.
Using this correlation, 4 Ollin (4 Quake) is 3 Etznab by the Mayan calendar; and in 2425 (or perhaps 2424, as vague years are slightly too short: although my source for this correlation based it in part on the Aztec calendar being tied to the Sun well enough that, unlike the Mayan calendar, it may have included a correction to the Xiuhpohualli giving it accuracy equivalent to that of the Julian calendar by adding 13 days after each calendar round) the dates of 3 Etznab are August 2, 2424 and April 19, 2425.
Another correlation for the Aztec calendar may be the one that will eventually prove popular as another opportunity for needless panic is sought once the Maya disappoint us on December 21, 2012; but having the end of the world in the year 2424, it must be admitted, gives tribute to Zager and Evans.
One thing, however, has not been checked in these calculations. Because the "Leyende de los Soles" is the only source for a date on which the previous Suns began and ended, it has not been checked if those years actually correspond to the end of a 52 year cycle according to the calendar correlation used here, so far, only for the Tonalpohualli. Checking the Xiuhpohualli, since the year 1519 corresponds to 1 Acatl, the fourteenth year of the cycle, then 1506 would be the first year of a cycle, followed by 1558, 1610, 1662, 1714 and 1766. This is seventeen years later than 1749, one of the end-of-cycle years that are a candidate for the end of the world according to the series from the Leyende de los Soles. This discrepancy would seem to allay any concerns about the dates identified here as presenting any real danger.
However, the Leyenda de los Soles presents the destruction at the end of a cycle as taking 13 years in one case, and 52 years in another, and Aztec belief had the human sacrifices taking place at the end of a calendar round to prevent the world from ending at any time during the next one. Thus, perhaps the world might end in the year 2424, but that end needs to be averted in the year 2389.
Also, a previous version of this page used a version of the correlation due to Gama where the year 1 Acatl began on December 31, 1518; so, November 8, 1519 was 9 Xochitl 16 Quecholli instead of 3 Tochtli 16 Quecholli; this led to the dates March 29, 2424, December 14, 2424 and August 31, 2425 for the end of the second 676 year period - and the date April 1, 2061 for the possible earlier date, the difference between the two correlations now being much larger than seven days.
It should also be noted that the dates given here are based on the assumption that the Tonalpohualli is absolutely continuous with the Aztecs as with the Maya. However, if the New Fire ceremony provides 13 intercalary days at the end of each calendar round, it appears that those dates were excluded from the Tonalpohualli as well as the Xiuhpohualli; Jacinto de la Serna was apparently the first to note this. This, of course, means that those intercalary days, if they did exist, do not leave an unmistakable footprint through the entire calendar, and in order to substantiate their existence, such things as documents giving dates in the calendar of Tenochtitlán for past eclipses would be required. Such records apparently do not exist, and thus at present the idea of such an intercalary period remains a surmise from indications that the Aztec calendar was tied to the actual solar year. The presence of such an intercalation was generally accepted by most scholars in 1845 - but by 1904, it was rejected as mere conjecture.
Using the 9 Quecholli correlation, the dates on which Aztec calendar rounds would begin in the two cases are:
Without intercalation: May 28, 1103 Jan 18, 1623 (Jul)/Jan 28, 1623 (Greg) May 15, 1155 Jan 5, 1675 (Jul)/Jan 15, 1675 (Greg) May 2, 1207 Dec 23, 1726 (Jul)/Jan 3, 1727 (Greg) Apr 19, 1259 Dec 21, 1778 Apr 6, 1311 Dec 7, 1830 Mar 24, 1363 Nov 24, 1882 Mar 11, 1415 Nov 20, 1934 Feb 26, 1467 Nov 7, 1986 Feb 13, 1519 Oct 25, 2038 Jan 31, 1571 Oct 12, 2090 With intercalation: Feb 13, 1103 Feb 13, 1623 (Jul)/Feb 23, 1623 (Greg) Feb 13, 1155 Feb 13, 1675 (Jul)/Feb 23, 1675 (Greg) Feb 13, 1207 Feb 13, 1727 (Jul)/Feb 24, 1727 (Greg) Feb 13, 1259 Feb 24, 1779 Feb 13, 1311 Feb 25, 1831 Feb 13, 1363 Feb 25, 1883 Feb 13, 1415 Feb 26, 1935 Feb 13, 1467 Feb 26, 1987 Feb 13, 1519 Feb 26, 2039 Feb 13, 1571 Feb 26, 2091
While November 7, 1986 was therefore the beginning of an Aztec calendar round by the most generally accepted correlation, which is not as universally accepted as the GMT correlation for the Mayan calendar (and it is known that among the Aztecs, different cities had their own starting points for the calendar), August 16, 1987 was apparently claimed to have ended one cycle, and August 17, 1987 to have begun a new one... these two days having comprised the famous Harmonic Convergence organized by José Argüelles.
And then there's the uncommon from Urza's Legacy of the same name.
The Kali Yuga is held to have begun on February 18, 3102 B.C. (Julian)... and is expected to last 432,000 years. So we can worry about the end of the world in 428,899 A.D. or thereabouts, by that standard.
December 21, 2012, of course, has come and gone.
But I have recently become aware of a prediction that, according to the Bible, the world is coming to an end on August 21, 2017.
The Bible, of course, says the following about the end of the world:
But of that day and hour knoweth no man, no, not the angels of heaven, but my Father only.
Matthew 24:36
Modern translations add, after "the angels of heaven", "nor the Son", and say "the Father" rather than "my Father". That Jesus did not know the date of the end of the world when He was in this world as a human being does not raise any issues, but this would seem to imply that, since only God the Father knows the date of the end of the world, even the Holy Spirit did not know that date, at least when Jesus spoke those words.
This would seem to conflict with at least a simplistic understanding of the Trinity.
But the Bible also says:
And when ye shall see Jerusalem compassed with armies, then know that the desolation thereof is nigh. - Luke 21:20
So likewise ye, when ye see these things come to pass, know ye that the kingdom of God is nigh at hand. Verily I say unto you, this generation shall not pass away, till all be fulfilled. - Luke 21:30-31
So, while it may be impossible to set an exact date, the possibility of narrowing down the time of the end of the world to a human lifespan is not excluded. The group of verses from which I quoted do create one problem; the destruction of the Temple in Jerusalem, matching the description in this prophecy, took place in 79 AD, and, of course, the end of the world did not happen within a normal human lifespan of that date.
But if we simply take the destruction of the Temple as a type of the end-time tribulations, that issue can be put aside.
Still, the Bible says:
And, behold, I come quickly; and my reward is with me, to give every man according as his work shall be. - Revelation 22:12
Two thousand years doesn't seem very quick. One would think that an absolute upper limit to "quick" would be a human lifetime. And the Bible says:
The days of our years are threescore years and ten; and if by reason of strength they be fourscore years, yet is their strength labour and sorrow; for it is soon cut off, and we fly away. - Psalms 90:10
So there is a Biblical value for the length of a human life, 70 years.
What is the key that can make sense of Bible end-times prophecy? How about:
For a thousand years in thy sight are but as yesterday when it is past, and as a watch in the night. - Psalms 90:4
But beloved, be not ignorant of this one thing, that one day is with the Lord as a thousand years, and a thousand years as one day. - 2 Peter 3:8
This simply describes one of the attributes of God's omnipotence, and of His eternal nature, of course, and there are no genuinely solid grounds for using it to time Bible prophecy. But people seeking to extract a date for the end of the world have done as bad or worse.
How many days are there in 70 years? 25,550 days. So "clearly" the Bible reassures us that the end of the world, though it could come tomorrow, will happen no later than 25,550,079 A.D.! Which is, at least, much sooner than the Sun's departure from the Main Sequence.
While little is definitely known about the calendar used by the Inca, some hypotheses as to its nature show it as much simpler than those based on the Mayan cyclic calendar.
The Inca may have had a solar calendar of 365 days, to which one day was added by observation at the end of the year when required. The year began on the winter solstice (which would have been the summer solstice in the southern hemisphere, where Peru is located) with the "great festival" Caypac Raymi.
Also, a lunar calendar of 328 days, with an 8-day week, may have been used; again, the source claims that one day was added when needed by observation. Given that the lunar month is 29.530588853 days, and 11 lunar months are about 324.8 days, it is unclear what was being observed. In fact, the discrepancy between the actual lunar month and 29 1/2 days adds up to almost exactly three days in every 98 lunar months; how to simply deal with this may be an interesting question.
Another source has the Inca numbering the years by an era, after the same fashion as done in the West; the year 2000 would have been the year 5519 in the Inca calendar.
Returning, now, to the Mayan calendar, the Tzolkin and the Haab calendars are the most well-known parts of the Mayan calendrical system, as they were also adopted by the Aztecs. The Long Count is the next most well-known part. Long Count dates were written using a system of positional notation for numbers which included a symbol for zero, in which a dot stood for one and a bar stood for five within the symbols for digits from 1 through 19. But Mayan inscriptions of dates often consisted of a Long Count date, a Tzolkin date, and then a group of glyphs called the Lunar Series, and finally the Haab date.
The decipherment of the glyphs in the Lunar Series took place only recently, and I present an attempt to describe them briefly.
As not all inscriptions including the Lunar Series included all the glyphs, the designations they were given by archeologists, in order, are G, F, Z, Y, E, D, C, X, B, and A.
Glyph G indicates one of nine lords of the underworld; the series of nine is synchronized with the last two digits of the Long Count. Glyph F is simply a marker for glyph G.
The glyphs Z and Y together (in a somewhat complicated manner) the position of the day in a regular succession of seven days, just like the days of our own week. This seven-day cycle, in combination with the nine-day cycle indicated by glyph G, and the 13-day cycle in the Tzolkin calendar, produced an 819-day cycle.
Glyph E is the first vigesimal digit, and glyph D the second vigesimal digit, of the Moon's age. Although some have suggested that the Maya reckoned the Moon's age from the full Moon, the GMT correlation is consistent with it being reckoned from the new Moon.
Glyph C indicates the place of the current lunation in a recurring cycle of 18 lunations.
Glyph X indicates the place of the current lunation in a cycle of either 6 lunations or 5 lunations. The rule for this sequence was changed at different times in Mayan history; originally, the cycle was always 6 lunations long, then it changed to one that seems to have been either 6 lunations or 5 lunations in length with equal frequency. A mixture of 6 lunation cycles with only a few 5 lunation cycles would have been useful in predicting eclipses, and so it appears that this was not the purpose of glyphs C and X. But the value of glyph X usually correlates with whehter the current lunation is 29 or 30 days long (as glyph A indicates).
Glyph B appears to be a marker for glyphs C and X which takes different forms due to grammatical rules.
Glyph A indicates whether the current lunation is 29 days long or 30 days long.
The age of the Moon was not calculated numerically in a fashion that did not alter over the life of the Mayan calendar. But it does not appear to have been derived from observation alone, either. Instead, 29-day months and 30-day months usually alternated. One study has led to the conclusion that during the classical period, the Maya used a cycle in which 81 lunar months included 38 months of 29 days and 43 months of 30 days. In Copan, the capital of one Mayan kingdon, it appears that a cycle of 149 lunar months with 70 months of 29 days and 79 months of 30 days was used instead.
While the Aztecs are said to have mainly used only the Haab and Tzolkin portions of the calendar, they did give names to the Nine Lords of the Night, and only their names, rather than those used by the Maya, are today known with confidence:
1) Xiuhtecuhtli 2) Iztli 3) Pilcintecuhtli 4) Cinteotl 5) Mictlantecuhtli 6) Chalchiuhtlicue 7) Tlazolteotl 8) Tepeyollotl 0,9) Tlaloc
and a perpetual calendar for this part of the cycle can be constructed:
1) Tll Xiu Izt Pil Cin Mic Cha Tlz Tep
2) Xiu Izt Pil Cin Mic Cha Tlz Tep Tll
3) Izt Pil Cin Mic Cha Tlz Tep Tll Xiu
4) Pil Cin Mic Cha Tlz Tep Tll Xiu Izt
5) Cin Mic Cha Tlz Tep Tll Xiu Izt Pil
6) Mic Cha Tlz Tep Tll Xiu Izt Pil Cin
7) Cha Tlz Tep Tll Xiu Izt Pil Cin Mic
8) Tlz Tep Tll Xiu Izt Pil Cin Mic Cha
9) Tep Tll Xiu Izt Pil Cin Mic Cha Tlz
MAR 1 2 3 4 5 6 7 8 9
10 11 12 13 14 15 16 17 18
19 20 21 22 23 24 25 26 27
28 29 30 31
APR 1 2 3 4 5
6 7 8 9 10 11 12 13 14
15 16 17 18 19 20 21 22 23
24 25 26 27 28 29 30
MAY 1 2
3 4 5 6 7 8 9 10 11
12 13 14 15 16 17 18 19 20
21 22 23 24 25 26 27 28 29
30 31
JUN 1 2 3 4 5 6 7
8 9 10 11 12 13 14 15 16
17 18 19 20 21 22 23 24 25
26 27 28 29 30
JUL 1 2 3 4
5 6 7 8 9 10 11 12 13
14 15 16 17 18 19 20 21 22
23 24 25 26 27 28 29 30 31
AUG 1 2 3 4 5 6 7 8 9
10 11 12 13 14 15 16 17 18
19 20 21 22 23 24 25 26 27
28 29 30 31
SEP 1 2 3 4 5
6 7 8 9 10 11 12 13 14
15 16 17 18 19 20 21 22 23
24 25 26 27 28 29 30
OCT 1 2
3 4 5 6 7 8 9 10 11
12 13 14 15 16 17 18 19 20
21 22 23 24 25 26 27 28 29
30 31
NOV 1 2 3 4 5 6 7
8 9 10 11 12 13 14 15 16
17 18 19 20 21 22 23 24 25
26 27 28 29 30
DEC 1 2 3 4
5 6 7 8 9 10 11 12 13
14 15 16 17 18 19 20 21 22
23 24 25 26 27 28 29 30 31
JAN 1 2 3 4 5 6 7 8 9
10 11 12 13 14 15 16 17 18
19 20 21 22 23 24 25 26 27
28 29 30 31
FEB 1 2 3 4 5
6 7 8 9 10 11 12 13 14
15 16 17 18 19 20 21 22 23
24 25 26 27 28 29
with the table indicating which row at the top to use for each year of the century having this form:
Gregorian
6 2 7 3 8 4 9 5 1 1600 2000 2400 2800 3200 3600
8 4 9 5 1 6 2 7 3 1700 2100 2500 2900 3300 3700
1 6 2 7 3 8 4 9 5 1800 2200 2600 3000 3400 3900
3 8 4 9 5 1 6 2 7 1500 1900 2300 2700 3100 3500 4000
Julian
1 6 2 7 3 8 4 9 5 200 500 800 1100 1400 1700
4 9 5 1 6 2 7 3 8 0 300 600 900 1200 1500
7 3 8 4 9 5 1 6 2 100 400 700 1000 1300 1600
00 01 02 03 04 05 06
07 08 09 10 11
12 13 14 15 16 17 18
19 20 21 22 23
24 25 26 27 28 29 30
31 32 33 34 35
36 37 38 39 40 41 42
43 44 45 46 47
48 49 50 51 52 53 54
55 56 57 58 59
60 61 62 63 64 65 66
67 68 69 70 71
72 73 74 75 76 77 78
79 80 81 82 83
84 85 86 87 88 89 90
91 92 93 94 95
96 97 98 99
For years B.C. in the Julian calendar, the following chart is the one to use:
2 7 3 8 4 9 5 1 6 3399 3099 2799 2499 2199 1899 1599 1299 999 699 399 99
5 1 6 2 7 3 8 4 9 3299 2999 2699 2399 2099 1799 1499 1199 899 599 299
8 4 9 5 1 6 2 7 3 3199 2899 2599 2299 1999 1699 1399 1099 799 499 199
99 98 97 96 95 94
93 92 91 90 89 88
87 86 85 84 83 82
81 80 79 78 77 76
75 74 73 72 71 70
69 68 67 66 65 64
63 62 61 60 59 58
57 56 55 54 53 52
51 50 49 48 47 46
45 44 43 42 41 40
39 38 37 36 35 34
33 32 31 30 29 28
27 26 25 24 23 22
21 20 19 18 17 16
15 14 13 12 11 10
09 08 07 06 05 04
03 02 01 00
As for the seven lords of the Earth, again based on the correlation of 0.0.0.0.0 to September 6, 3114 B.C., just as, since that date is 4 Ahau 8 Cumhu, the 13-day cycle started three days earlier, on September 3, 3114 B.C., that date being 1 Caban 5 Cumhu, the 7-day and 9-day cycles also start on that date. September 3, 3114 B.C. was a Friday, so that is the day ruled by the first lord of the Earth.
Because the Maya are known to have made incredible achievements in their tracking of the motions of the heavenly bodies, some extravagant claims have been made concerning their achievements. Thus, some have claimed that the 260-day Tzolkin cycle reflects an inner harmony of the entire Solar System, and might attribute it to a supernatural or extraterrestrial source.
One such claim is that despite using a 365-day vague year, the Maya actually knew the length of the tropical year to a greater accuracy than that reflected even in the Gregorian calendar. This is based on several inscriptions referring to intervals of 1,508 Haab; such an interval corresponds almost exactly to 1,507 tropical years.
Although, as recorded in the Dresden Codex, the Maya did work out some remarkable relationships between the phases of the Moon and the synodic period of Venus with the Haab and the Tzolkin, the general consensus is that the 260-day Tzolkin was not designed as a way to produce these relationships, but was simply a pre-existing period used for purposes of divination and established before the flowering of Mayan astronomical achievement.
It may also be noted that the orbit of Venus around the Sun takes 224.701 days, and its synodic period (the average time between recurrences of a given relationship between the Earth and Venus around the Sun, such as maximum elongations) is 583.92 days. Any simple connection between either of those periods and the 260-day Tzolkin cycle is not immediately obvious.
However, 584 days is a multiple of 4 days, and so it is not relatively prime to the 20-day cycle. Thus, 65 Venus synodic periods of 584 days will correspond to 146 Tzolkin cycles of 260 days. This, in itself, is not remarkable; any two cycles will have a least common multiple.
The Haab and Tzolkin calendars synchronize every 52 Haab years of 365 days, and every 73 Tzolkin cycles of 260 days, this being the Calendar Round. As 73 is half of 146, two calendar rounds do lead to a recurrence of the 584-day synodic period of Venus, and this is, at the least, a coincidence.
But perhaps not as big a coincidence as it might seem. 104 is a multiple of eight, 52 being four times thirteen as we know from playing cards, and the positions of the planet Venus in the sky very nearly repeat themselves every eight years.
In about 2,922 days, the Earth orbits the Sun eight times, and in about 2,921 days, Venus orbits the Sun thirteen times, thus the Earth overtakes Venus five times in this span, but the positions of Venus change slightly from one eight-year period to the next. Five times 584 days gives 2,920 days, eight times the vague year of 365 days, so the Haab, rather than the Tzolkin, might be considered as being tied to the planet Venus, even though, as a year, it is obviously also tied to the Sun.
The Maya were aware of the synodic cycle of Venus, and did use 584 days as an approximation to its duration. The Dresden Codex, one of the few surviving Mayan books, includes tables of the position of Venus. What is unclear, however, is that this cycle formed the basis of their Tzolkin calendar cycle. The Lunar Series glyphs found in many inscriptions include the day of the lunar month and the position of the day in both a 7-day week and a 9-day week, but they do not give an indication of the position of the day within the 584 day cycle either.
One element of the well-known parts of the Mayan calendar that might be affected by Venus, however, is the Haab portion. Eight years are also approximately five synodic periods of Venus. Five times 584 days is 2,920 days, which is also eight times 365 days. Thus, the omission of the leap year could be credited to Venus; the only contribution made by the 260-day cycle is that 260 is divisible by 4, which allows two calendar rounds to be a multiple of eight vague years.
There is some evidence from monuments, but not of a conclusive nature, that suggests the Maya might have been aware that 29 calendar rounds of 52 Haab each amounted not only to 1,508 Haab, but to 1,507 tropical years, leading to an approximation to the tropical year of about 365.24220305 days, much closer than that used in our present Gregorian calendar.
Adding 13 days to each calendar round with a New Fire ceremony, to lead to a calendar equivalent to the Julian calendar, would lead to 29 calendar rounds adding 377 days to 1,508 Haab, or about 12 days too many. Approximating 1,508 tropical years by 1,508 Haab and 365 days - or 1,509 Haab - leads to a value of about 365.242 days for the tropical year, and so an Aztec-style alternative is available, with a cycle of 17 long calendar rounds each with 13 extra days, and 12 short calendar rounds each with 12 extra days, but this no longer offers a significant improvement on the Gregorian calendar: given that the tropical year is 365.2422 days long, the Gregorian year 365.2425 days, and this approximation to the tropical year 365.2420 days, the error is merely reduced from .0003 days to .0002 days in the opposite direction; it would be better to combine the two calendars somehow than to go to the trouble of switching calendars for only a slight improvement.
Thus, rather than modifying the Mayan calendar itself by adding leap days, it needs to be kept intact, with a seasonal calendar tied to it, so as to achieve the correct 1507:1508 ratio instead of the less accurate 1508:1509 ratio.
So the goal is to have 365 leap days occurring within the seasonal years that go by in parallel to 29 calendar rounds.
One pattern which could achieve this is:
JSJSJSJJSJSJJSXSJJSJSJJSJSJSJ
J would stand for one of 16 periods of 52 years in which 13 of those years, one of every four, was a leap year.
S would stand for one of 12 periods of 52 years with only 12 leap years. They could be organized with leap years at intervals of 4, 5, and 4 years rather than simply omitting one leap year the way the Gregorian calendar does.
X stands for a period of 51 years with 13 leap years. That could be done by having 3 years between leap years instead of four once.
Then, to make the succession of leap years more regular, in the middle of the X, have a 5 year period between leap years instead, and pull the leap years away by shortening one interval of 5 years to 4 years in each adjacent S. (If the leap day was moved a month earlier within each S, then each S would instead be otherwise identical; that would be another way to shorten the cycle by a year.)
The next step would be to make the succession more even by migrating some 5 year intervals from the S rounds to the adjacent J rounds.
This is another version of the Sothic cycle of the ancient Egyptians, in which 1,460 Julian years are equal to 1,461 vague years.
Also, the synodic period of Mars is 779.94 days. 780 days is 260 days times three. Thus, if anything, the 365-day period is both a Sun year and a Venus year, and the 260-day period is a Mars year rather than a Venus year as some have called it. Because of the limited amount of surviving records, we cannot be certain that the Maya kept track of Mars, or of Jupiter, Saturn, and Mercury.
That there would be cycles available to them for this, however, is true:
Tropical year 365.242199 1,507 * 365.2422 = 1,508 * 365 = 2,117 * 260
Synodic month 29.53059 405 * 29.53086 = 46 * 260
Synodic periods of:
Mercury 115.88 9 * 115.56 = 4 * 260
7 * 117 = 819
22 * 116.14 = 7 * 365
Venus 583.92 5 * 584 = 8 * 365
61 * 583.93 = 137 * 260
Mars 779.94 780 = 3 * 260
Jupiter 398.88 39 * 399 = 19 * 819
140 * 398.89 = 153 * 365
88 * 398.86 = 135 * 260
Saturn 378.09 13 * 378 = 6 * 819
We have seen above that the 819-day cycle was formed by taking the 13-day week of the Tzolkin, and combining it with a 9-day week indicating the lords of the night and a 7-day week indicating the lords of the earth; this cycle was used for divination of the affairs of kings even as the 260-day cycle was used for divination by the ordinary people, and it could be interpreted as relating to the motions of Jupiter and Saturn.
In addition to a relationship between the synodic period of Mercury and the Tzolkin, a less accurate one to the Haab is also shown that illustrates the curious fact that the year is about pi times the synodic period of Mercury.
Possible relationships between the synodic periods of Venus and Jupiter to the 260-day Tzolkin are shown that have been used as part of the basis for a claim that this period had relationships to all the Solar System cycles. However, continued fraction methods could obtain equally good relationships for almost any other number of days.
After three Saros cycles, also known as an exeligmos, which amounts to 19,756 days, eclipses will tend to recur in the same geographical location. This is only four days short of 19,760 days, which is 76 Tzolkin cycles of 260 days. Thus, it is possible to claim that the Tzolkin cycle somewhat simplifies the calculation of eclipses as well, an odd four days each cycle being relatively easy to calculate. However, the Saros cycle does not appear to have been used by the Maya.
The Dresden Codex also shows that the Maya used an approximation of the lunar month in which 81 lunar months were 2,392 days long. Repeating this approximation five times obtains 405 lunar months, and 11,960 days, with an accumulated error of about 1/9th of a day by which the 405 lunar months are shorter than 11,960 days, and 46 Tzolkin cycles of 260 days.
The lunar tables in the Dresden Codex divided the lunations into groups of 6 lunations which are usually 177 days long, but occasionally 178 days long, and the occasional group of 5 lunations which is 148 days long. This was done to predict days on which an eclipse was possible.
If one slides this sequence of 11,960 days against itself at a displacement of 6,585 days, a Saros cycle being 6,585 and a third days, one will find the two sequences usually match; this is a natural consequence of the fact that it is intended to keep track of eclipses. But the sequence was not restarted after 6,585 days or 19,756 days, but at its end, 11,960 days, and so the Saros cycle was not what was used by the Maya; they were less accurate in predicting eclipses than the ancient Greeks as far as we know.
The 405 lunation cycle, in addition to being five times a cycle of 81 lunar months in 2,392 days, is also three times a cycle of 135 lunations called the Tritos. This is the next closest approximation to a coincidence between the synodic month and half of the draconic month after the Saros; beyond the Saros, the Inex, of 358 lunations, is an even closer approximation to the Saros to the relationship between those two cycles.
Ideally, then, a table of 405 lunations should repeat the exact same pattern of alternating 29 and 30 day lunations five times, and the same pattern of 6-lunation and 5-lunation eclipse semesters six times. The one in the Dresden Codex did not indicate the length of individual lunations, and the pattern of eclipse semesters was nearly, but not exactly, repeated.
135 lunations would include twenty semesters of six lunations and three semesters of five lunations. In the Dresden codex, all the semesters of five lunations are 148 days long. Of the twenty semesters of six lunations, eighteen are 177 days long, and two are 178 days long.
2,392 days would include 43 lunations of 30 days, and 38 lunations of 29 days.
How closely could one work these two cycles together?
Because the five lunation semester is fully consistent with alternation of 29 and 30 day lunations, its location isn't constrained by the distribution of pairs of 30 day lunations, except to avoid one within such a short semester, so an even distribution should be possible (the one in the Dresden Codex was not as even as possible).
81 lunations, consisting of 38 pairs of one 30-day lunation and one 29-day lunation, plus 5 extra 30-day lunations to distribute evenly, could follow this pattern:
8*(30 29) 30 7*(30 29) 30 8*(30 29) 30 7*(30 29) 30 8*(30 29) 30
If we impose three repetitions of a maximally-regular repetition of an alternation between five and six lunation eclipse semesters on five repetitions of that sequence, we may have to make some slight adjustments to fit within the assortment of semester types shown above. Of course, other sequences of three 30-day months and two 29-day months could produce a 148-day eclipse semester.
A regular pattern of semesters might be like this:
7*(6) 5 6*(6) 5 7*(6) 5
Let us assume we are dealing with twelve types of eclipse semesters:
29 30 29 30 29 30 177 A 29 30 29 30 30 29 177a B 29 30 30 29 30 29 177b C 30 29 30 29 30 29 177c D 29 30 29 30 30 148a 1 29 30 30 29 30 148b 2 30 29 30 29 30 148 3 30 29 30 30 29 148c 4 30 30 29 30 29 148d 5 30 29 30 29 30 30 178 x 30 29 30 30 29 30 178a y 30 30 29 30 29 30 178b z
With these types of eclipse semesters, we would be able to build stretches of 29 and 30 day lunations, with occasional flips in the parity of the sequence involving two 30-day lunations in a row, so we have flexibility with which to at least approximate the regular sequences above.
Since five lunations in 148 days lead to three 30-day lunations and only two 29-day lunations, it's easy to associate a pair of consecutive 30-day lunations with a short semester. This can also be avoided, but then the six-lunation semester before must end, and the six-lunation semester after must begin, with a 29-day lunation. Meeting this constraint while including only two 178-day periods of six lunations in a 135-lunation Tritos will be the challenge.
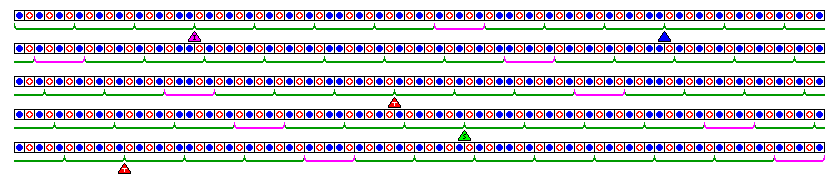
Taking the regular pattern of five and six lunation semesters and placing it against the regular pattern of 29 and 30 day lunations, the following image shows one possible sequence:

This sequence includes some 147-day five lunation semesters, unlike the sequence given in the Dresden Codex, and, as noted, that sequence was not as exactly regular as this one.
But much of the sequence fits into the plan of possible semesters shown above. As well, while the succession of 29 and 30 day lunations follows the actual lengths of lunations closely, and thus shouldn't be altered, because the purpose of the semesters is to indicate the days that have a higher chance of an eclipse, they could be altered. Since a sequence of six-lunation and five-lunation semesters only approximates the relation between the synodic month and the draconic month, eclipses would not be likely at the parts of that sequence which were furthest out of step with a semester of uniform size, just a bit less than six lunations.
If the cycle is started so that the midpoint of the sequence of semesters coincides with a full moon (if it is lunar eclipses that are being checked for) that is exactly on the ecliptic, then the start and end days of a five-lunation semester will be those where the full moon is furthest from the ecliptic, and thus the short semester could be moved. In the diagram above, in the three cases where we would wish to do so, we would also be able to eliminate an adjacent 178-day semester by doing so.
The diagram above shows a blue triangle at one such midpoint; red triangles indicate successive Tritos cycles after that point, a green triangle indicates one Saros cycle after that point, and a purple triangle indicates an Inex cycle; because the blue triangle does not begin the chart, it is placed where it would be on another repetition of the diagram. As the Saros and Inex cycles are more accurate than the Tritos, they show places where a new repetition might be shifted to as the sequence loses synchronization.
If we make the adjustements needed to avoid 147-day semesters, the resulting sequence becomes:
..!....*..!...*....!..*....!..*....!.oO....oO!......oO......*..!....*
where * and o stand for 148-day semesters, . and O stand for 177-day semesters, and ! stands for a 177-day semester. The symbols o and O are used where a five-lunation semester was moved to avoid it being 147 days in length.
The actual sequence in the Dresden Codex is:
...*...!.....*!...*.......*..!......*!...*.......*..!.....*.!...*....
The second 148-day semester out of every three is considerably delayed, and the 178-day semesters are more evenly distributed.
Can the even distribution of 29-day and 30-day lunations above be made to correspond with this sequence?
For a first attempt, one might try and slide the sequence of semesters along so as to fit as closely as possible:
Dresden: ...*...!.....*!...*.......*..!......*!...*.......*..!.....*.!...*....
Regular: ...*..!...*....!..*....!..*....!.oO....oO!......oO......*..!....*..!.
!..*....!.oO....oO!......oO......*..!....*..!....*..!...*....!..*....
..oO......*..!....*..!....*..!...*....!..*....!..*....!.oO....oO!....
To break the Dresden Codex sequence into five aligned pieces, so as to determine possible locations for pairs of 30-day lunations, one would have to expand the semesters into individual lunations. So, * becomes *****, ! becomes !!!!!!, each showing a stretch with an excess 30-day lunation, and . would alternate between ...... and ======, since a pair of 30-day lunations could also be placed at the boundary between two normal semesters.
That gives us this sequence:
......======......*****......======......!!!!!!......======......======......**** *!!!!!!......======......*****......======......======......======......*****.... ..======!!!!!!......======......======......======*****!!!!!!......======......** ***......======......======......======......*****......======!!!!!!......======. .....======......*****......!!!!!!......======......*****......======......======
One thing we can do to solve for the location of 30 and 29 day locations is to note the cases where a 178-day semester overlaps a 177-day semester with an offset of only one lunation. In each such case, a 30-day lunation must be given up, and a 29-day lunation gained, when going from the 178-day semester to the 177-day semester. This should allow us to quickly determine if a consistent sequence of 81 lunations always repeating is even possible. There are no cases of an exact overlap of a 178-day semester with a 177-day semester, making such a solution obviously impossible.
......======......*****......======......!!!!!!......======......======......**** *!!!!!!......======......*****......======......======......======......*****.... ..======!!!!!!......======......======......======*****!!!!!!......======......** ***......======......======......======......*****......======!!!!!!......======. .....======......*****......!!!!!!......======......*****......======......====== SL LSL LS SL LS SL LS SL LSL LS
Our immediate deductions give us, because of the way in which the 178-day semesters are scattered over the 81-lunation cycle, six places in that cycle where there must be a pair of 30-day lunations; since we only have five such pairs to distribute, we can conclude that the series of semesters in the Dresden Codex is not consistent with any alignment of a maximally-regular distribution of 29 and 30 day lunations.
......======......*****......======......!!!!!!......======......======......****
*!!!!!!......======......*****......======......======......======......*****....
..======!!!!!!......======......======......======*****!!!!!!......======......**
***......======......======......======......*****......======!!!!!!......======.
.....======......*****......!!!!!!......======......*****......======......======
SL LSL LS SL LS SL LS SL LSL LS
!!!! !!!! !!!! !!!! !!!! !!!!
......======......*****......======......!!!!!!......======......======......****
*!!!!!!......======......*****......======......======......======......*****....
..!!!!!!======......======......======......======*****!!!!!!......======......**
***......======......======......======......*****......!!!!!!======......======.
.....======......*****......!!!!!!......======......*****......======......======
S L L S SL LS SL LS S L L S
!!!! !!!!
......======......*****......======......!!!!!!......======......======......****
*!!!!!!......======......*****......======......======......======......*****....
..======!!!!!!......======......======......======*****!!!!!!......======......**
***......======......======......======......*****!!!!!!......======......======.
.....======......*****......!!!!!!......======......*****......======......======
SL LSL LS SL LS SL LS SL LS
!!!! !!!! !!!! !!!! !!!!
An attempt to solve this by moving two 178-day semesters by one place leads to places where, because two 29-day lunations in a row are impossible, pairs of 30-day lunations are now fixed in locations that are too close together.
Moving one 178-day semester by two places allows it to be placed where there are no overlaps of the type used to make the initial deductions. An overlap with a 148-day semester implies a 30-day lunation that is already indicated for other reasons.
This would seem to allow a recurrent sequence, even if not one that is maximally regular. However, there is also one case where two 148-day semesters overlap with an offset of one semester. As this means what is given up must be of the same type as what is gained, this again forces a sixth location for two consecutive 30-day lunations.
The Mayan calendar also included an 819-day cycle. It's purpose had been mysterious, for the most part, until recently.
It had already been known that 819 days was approximately seven times the synodic period of Mercury.
What John Linden and Victoria Bricker recently discovered was that this cycle also related to the synodic periods of the other planets visible to the naked eye, if one considered somewhat larger multiples of the cycle, and so it facilitated calculating the phenomena of the various planets.
Of course, if one takes enough multiples of any cycle, one usually could force correspondences, which is why no one had previously discovered that taking a reasonable number of cycles for the 819-day cycle would work.
Planet Synodic Period Multiples of the Synodic Multiples of the
(days) Periods 819-day Cycles
Mercury 115.88 115.88 * 7 = 811.6 1 * 819 = 819
Venus 583.92 583.92 * 7 = 4,087.44 5 * 819 = 4,095
Mars 779.94 779.94 * 21 = 16,387.74 20 * 819 = 16,380
Jupiter 398.88 398.88 * 39 = 15,556.32 19 * 819 = 15,561
Saturn 378.09 378.09 * 13 = 4,915.7 6 * 819 = 4,914
Thus, a table of planetary positions organized by the 819-day cycle would only need a small correction from one repeat to the next.
The end of the world did not happen on schedule on December 21, 2012 A. D.. However, there is another case where the end of a world, in a minor way, did happen just when a calendar ran out!
Year code:
0 1 2 3 4 5 6 7 8 9
---------------------
1950 A B C D E F G H I J
1960 K L M N O P Q R S T
1970 U V W X Y Z
Month code:
A January G July
B February H August
C March I September
D April J October
E May K November
F June L December
This is the scheme of two-letter date codes that were stamped on Hemmi slide rules starting from at least 1951. By 1975, the rise of the pocket calculator spared Hemmi from figuring out what to do about representing later years in the absence of a letter of the alphabet after Z.
Magic: the Gathering, and the names of the expansion sets referenced here, as well as the card names, the mana symbols, and the pentagon of colors, are trademarks of Wizards of the Coast LLC, a subsidiary of Hasbro, Inc..