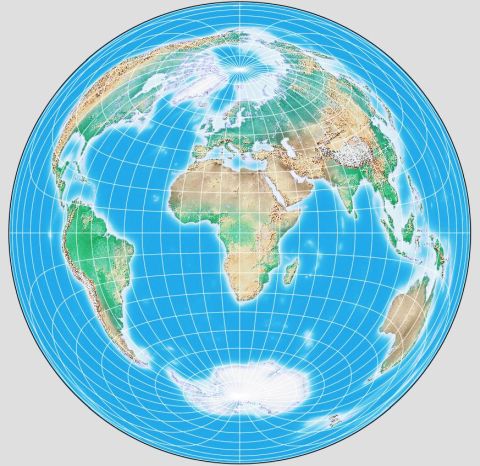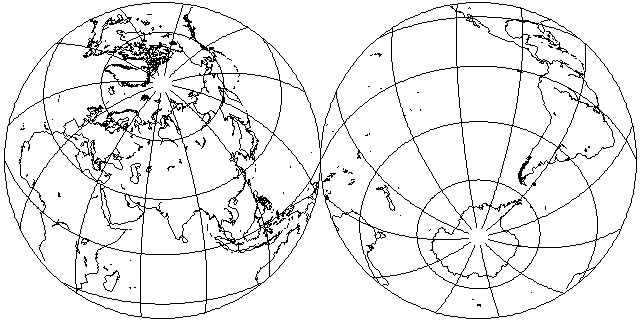
This map projection is one of the most popular projections used in atlases today.

Here, the center of the projection was chosen so that one hemisphere would contain a good representation of the Eurasian landmass, with a slight bias towards putting Europe near the center of the projection.
This projection is not usually used for hemispherical maps, but these hemispheres in the equatorial case illustrate the behavior of this projection:
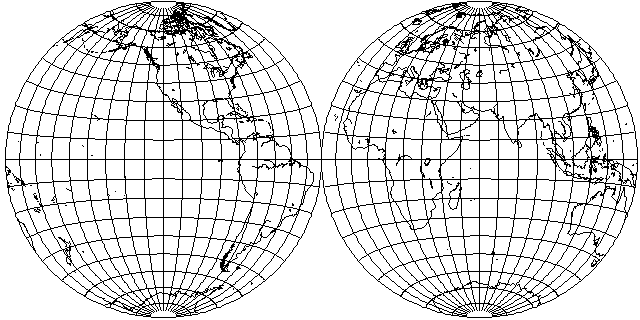
Because it is azimuthal, it is well suited to mapping regions that do not have any large difference between their north-south extent and their east-west extent. It is also equal-area; in the past, conformality was considered perhaps the most important property of maps, but today the fashion has swung in favor of equivalence, the property of being equal-area.
Conformal maps are well suited to showing wind and ocean currents, because at each point they show the angles through which things turn. They are also good for looking closely at a small area on the map, since each small area is well mapped, even if at a different scale than other parts of the map. Equivalent maps, on the other hand, are the appropriate ones to show densities and distributions; thus, they have taken on greater importance in our modern world in which statistics play a larger role.
The formula for the construction of the Lambert Azimuthal Equal-Area projection is quite surprising:
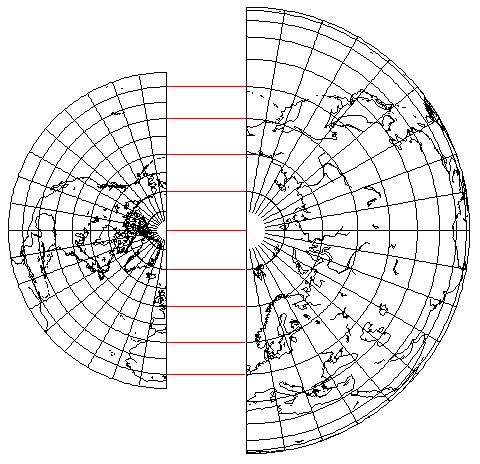
As this diagram showing a half from a hemisphere each of the Lambert Azimuthal Equal-Area Projection and the Orthographic Projection illustrates, if one just divides the angle away from the pole by 2, then otherwise the formula for the Orthographic projection gives the Lambert Azimuthal Equal-Area projection.
It might have been easier to suspect this relationship if one had the opportunity to see more often this projection applied to the entire globe, as shown here thanks to G.Projector: