On a previous page in this section, we dealt with a relatively little-known English unit of mass, the troy ounce.
Now, we will look at the values of some other relatively little-known English units. When the height of a horse is given in hands, each hand is 4 inches. In Britain, people's weights were usually quoted in stone rather than pounds; one stone is 14 pounds. Also, in the Troy system of weights, 24 grains make a pennyweight, with 20 pennyweights making up a troy ounce; in Apothecaries' weight, the grain and the troy ounce are still used, but now 20 grains make a scruple, 3 scruples (or 60 grains) make a dram, and there are 8 drams to the troy ounce.
Even more obscure is the division of the inch into 3 barleycorns, or 12 lines. With 12 lines to the inch, and 12 inches to the foot, lines were sometimes indicated with a triple prime, just as the third, one-sixtieth of a second, in angular measure was indicated.
Although they are available in most reference works, also little-known are the fathom (6 feet or 2 yards), the rod (5 1/2 yards, or 16 1/2 feet), the chain (66 feet, 22 yards, or 4 rods), and the furlong (660 feet, 220 yards, 10 chains or 40 rods), these latter units composing the mile, which is eight furlongs in length. A league is three miles long.
It might also be noted that while an acre is unusual for a unit of area in not being a square something-or-other, an acre is equal to ten square chains, and a square furlong is equal to ten acres. A unit used for measuring nuclear cross section, the barn, is 10^-28 square meters, or 10^-24 square centimeters, or 10^-22 square millimeters. Before looking it up, I had thought I had seen the figure 10^-15 relating it to a conventional area measure, which would have led nicely to the riddle "Why is a barn like an acre", but no such mistake was actually made in its definition. However, the millibarn and nanobarn are also used as units of cross-section, and these units are acre-like.
While the exact value of the historical cubit is variable, a cubit can be thought of as being roughly 18 inches in length, composed of 6 spans each 3 inches long, each span consisting of 4 digits each 3/4" wide. Three-quarters of an inch is also the horizontal spacing of keys on a typewriter.
Four cubits of 18 inches make a fathom of 6 feet; 100 fathoms make up the Greek stadium.
Of course, just as many countries had different feet, derived from the Roman foot, many countries had different miles.
But here I wish to speak of the nautical mile.
Nominally, the nautical mile is the length of a minute of latitude, thus making it simpler to relate distances in nautical miles to a ship's position in latitude and longitude.
As the Earth is not a perfect sphere, this was not an exact definition in itself, and also it had been desired to make the length of the nautical mile a round figure within existing systems of measurement.
Initially, given that the metre was originally defined as one ten-millionth part of the meridian of Paris from the North Pole to the Equator, it seemed logical to define the nautical mile as exactly 1852 metres on this basis. This was also adopted as the international definition of the nautical mile in 1929.
However, the United States defined the nautical mile as 6080.2 feet, and Britain defined the nautical mile as 6080 feet, as approximations of 1853.248 metres, derived from the Clarke ellipsoid of 1866.
In 1970, when Britain adopted the metric system, they changed their nautical mile to 1853 metres.
The circular slide rule portion of a dead reckoning flight computer includes a conversion between nautical and statute miles. I have seen different ratios used, and given the different values for the nautical mile, this can be sorted out:
76 to 66: from 5,280 feet to 6,080 feet exactly 1.15 to 1: from 5,280 feet to 6,072 feet or 1850.7456 metres
Another ancient foreign system of measure that is of some interest is that used in Japan.
When Japanese units were standardized in terms of metric units in 1891, the unit of length, the jo, was defined as 100/33 of a metre; the unit of volume, the sho, was defined as 2401/1331 of a litre, and the unit of mass, the momme, was defined as 15/4 of a gram.
There are ten shaku in a jo; ten sun in a shaku; ten bu in a sun, and ten rin in a bu. (The term bu is also a Japanese word for the fraction one-tenth, and rin also means one-hundredth.)
This is of importance today because the grid of a Go board is traditionally one shaku and four sun across, but one shaku and five sun long.
The spacing of the lines on the grid of a Go board is, of course, one-eighteenth the size of the whole board, and thus while it can be exactly expressed as a fraction, it cannot be so expressed as a decimal. This includes the thickness of the grid lines, of course, and thus the simplest calculation is based on the assumption that the outermost lines are the same thickness as the inner lines, and that the measurement of the size of the board is from the middle of the lines.
| Unit of measurement: | bu | millimeters | inches |
| Across | 140/18, or 7 7/9, or 7.777777... | 23 169/297, or 23.569023... | 0.9279143... |
| Along | 150/18, or 8 1/3, or 8.333333... | 25 25/99, or 25.252525... | 0.9941939... |
The lines on a Go board are about 1/25th of an inch, or one millimeter, in width. If we take this width to be, therefore, 0.3 rin, or 0.03 bu, and subtract it once in both directions, so that eighteen lines plus eighteen spaces are 1.3997 shaku across and 1.4997 shaku wide, we still do not get figures that divide exactly by eighteen in either direction.
Eighteen times 0.9941939... inches is about 17.89 inches.
For comparison, the American Checker Federation specifies that a checkerboard for tournament use is to have squares 2 inches on a side, making the board 16 inches long and wide. (The pieces are to be disks from 1 ¼" to 1 ½" in diameter.)
I have now come across another place where the traditional Japanese units of length are still used.
The size of a tatami mat is 1 9/11 metres by 10/11 of a metre, and so, since a jo is 100/33 of a metre, and a shaku is 10/33 of a metre, a tatami measures six shaku by three shaku. Floor space in Japanese homes is traditionally measured in terms of a unit of thirty-six square shaku, the square made by putting two tatami side by side.
Six shaku make up a unit of length in its own right, the ken.
The traditional Japanese units of length noted here, the bu and the shaku, correspond to traditional Chinese units of length. And the Chinese system of units of length has what could be called, in view of the topic of this section... a chequered history.
Recently, I happened to note some entries in a Chinese-English dictionary. A Ts'un was a Chinese inch, which was one-tenth of a Chinese foot. And a Chang was ten Chinese feet, and it was eleven feet, nine inches long, in English measure.
Looking up the article on Chinese units of length measure, though, while many different historical lengths for the Chinese foot (a Ch'in) were given, the length corresponding to the one indicated by that dictionary was not among them.
Some further research cleared up the mystery.
The particular length standard that served as the basis for the definitions in that dictionary, in which a Chinese foot would be 14.1 inches (358.14 mm) in length, was the one established for customs duties in China by the Treaty of Tientsin of 1859, but that was the only purpose for which that particular standard of length measure was used.
For about 500 years, the Chinese foot was about 231 millimetres long - from the latter part of the Eastern Chou (Zhou) dynasty through the Ch'in (Qin) and Han dynasties. Over the centuries, its length had been continually increasing. In 1915, a standard value of 320 millimetres was established, and then shortly after, a revised stanard of 1/3 of a metre was adopted.
In Hong Kong and Macao, the unit of length was somewhat larger, there, the Chinese foot was 14 5/8" (371.475 mm) long, or more; a wooden ruler from Hong Kong, showing a Chinese foot with the ten Chinese inches being divided in turn into tenths following the 14 5/8" standard is pictured in Wikipedia.
When the Ch'in was 231 mm in length, six Ch'in made one Pu, and 300 Pu made one Li, the Chinese unit performing a function similar to that of the mile or the kilometre; thus, the Chinese proverb was really "A journey of a thousand li begins with the first step".
Later, the Pu became only five Ch'in, and thus 360 Pu were required to make a Li, so that there would be the same number of Ch'in in a Li.
Because Taiwan was long under Japanese rule, the Taiwanese Ch'in was the same as the Japanese Shaku, 303.030303... mm, and the Taiwanese Ts'un was the same as the Japanese Sun, a tenth of that.
The USCF specifies that a chessboard as used in tournaments is to have squares from 2 inches on a side to 2 1/2 inches on a side, so that the length of the board may range from 16 inches to 20 inches. Note that 18 inches falls in the middle of this range.
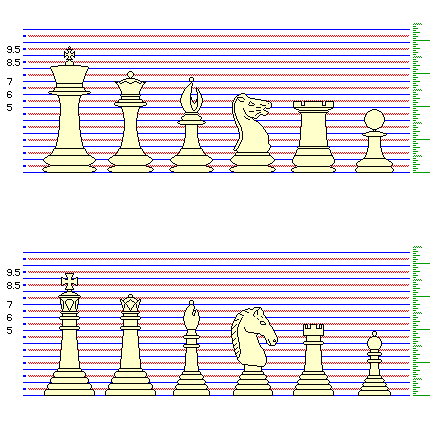
Chess pieces, of course, vary in size, the King being the largest and the Pawn the smallest. Since the size of a chess set is usually specified by the height of the King, a standard for the size of chess pieces for tournaments is also given: the King height should range from 3 3/8" to 4 ½", and the base of the King should be from 40% to 50% of its height.
The same proportion of base to height is given by FIDE; one web site claimed that FIDE specified a range of King heights from 8.5 cm to 10.5 cm, which is from just over 3 1/3" to just over 4 1/8", which leads to a FIDE size range that is centered about a smaller size of piece than the USCF range, although chess pieces with a King height from 3 ½" to 4" will be well within both ranges. The official FIDE web site simply says that the height of the King should be "about 9.5 cm", which is approximately 3 ¾".
Since I wrote that, I have found from FIDE a standard for equipment at events that does give more detail. The King height is to be 9.5 cm plus or minus 10%, which would be 8.55 cm to 10.45 cm.
But the standard also specifies sizes for the other pieces, rather than leaving that to chance:
King 9.5 cm Queen 8.5 cm Bishop 7 cm Knight 6 cm Rook 5.5 cm Pawn 5 cm
Each piece may vary from these guidelines by 10% either way, but it is required that the heights of the pieces in the set have that order. So making the Rooks taller than the Bishops as an exercise of originality is not permitted.
The following image illustrates how well some pre-existing chess set designs, the original Staunton pieces, and one Staunton-pattern plastic set of more recent vintage, correspond to these proportions:

I am surprised to hear that "chess pieces should be made of wood or plastic, or an imitation of these materials". Usually, plastic is thought of as an imitation of ivory. Of course, that material can hardly be recommended, given CITES regulations, but thinking of chess pieces being made of imitation plastic is for some reason difficult for me.
Still, I can quite understand that a list that includes, say, gutta-percha and the tagua nut might have been felt to be a waste of effort; organizers of chess tournaments aren't likely to use pieces carved from bone either.
Perhaps a more serious concern is that the standard also states "in all cases, boards should be rigid", which places many supposedly compliant inexpensive sets out of the running.
As for the size of the squares, their standard is from 5 cm to 6.5 cm, or just under 2 inches to just over 2 ½ inches, so this portion of the standard is essentially compatible.
The web site of one manufacturer of chess pieces notes that a chess board is well-matched to the size of the chess pieces used on it if the base of the King has a diameter which is 75% of the size of the square. Comparing this rule of thumb to the specifications noted above, the smallest possible King base is 67.5% of the size of the smallest square, and the largest possible King base is 90% of the size of the largest square.
This means both that the range of chess piece sizes is broader than that of board sizes, and that a board designed to be used both for chess tournaments and checker tournaments, with a set of chess pieces designed to go well with the board, is possible.
In the case of International Checkers, the Fédération Mondiale du Jeu de Dames does not define the size of the playing units in terms of exotic foreign units of measure such as shaku, or inches. Rather, the squares which make up the 10 by 10 board of this game are from 3.5 centimeters to 4.5 centimeters. The diameter of the checkers is to be from 7 to 14mm less than the size of a square, and their thickness is to be from 20% to 25% of their diameter. Also, they specify that a raised border of 5 mm to 6 mm is to surround the playing area of the board, which is quite an unusual requirement, unless the meaning is actually that the board itself is to have that thickness.
Thus, the board is from 35 centimeters to 45 centimeters in size; for comparison, 18 times 2.52525... centimeters is about 45.45 centimeters.
Since we have examined the game of Go, played on a board of 19 by 19 points and 18 by 18 squares, with the size of the board defined in the tradiditonal Japanese units of the bu and the shaku, and we have examined Chess, on the 8 by 8 board, and forms of Checkers on an 8 by 8 board and a 10 by 10 board, with both inches and centimeters used as units, and we have found that the size of the board always seems to be consistent, and in the neighborhood of 18 inches, it would seem to follow that wherever the adult human body is the same size, whatever system of units may be used, this is the size found to be most appropriate for a board used four tournament play.
The diameter of Mars at the Equator is now known to be about 6,786 kilometers; but if we took instead a value of 6,807.578 kilometers, this would give an equatorial circumference of 21,386.64 kilometers or 13,189.04 miles. Dividing this equatorial circumference into 360 parts would yield a karad of 36.914 miles; the haad, being the hundredth part of a karad, would then be 1,949.0592 feet in length, as it was given to be in the book A Fighting Man of Mars by Edgar Rice Burroughs.
In Thuvia, A Maid of Mars, an earlier book, an apparently erroneous figure of 2,339 feet is given, and this has been often quoted. However, if the error was in assuming that on Mars the circle is divided into 360 parts, as is the case on Earth, then it might be noted that if the karad were one 300th part of the equatorial circumference of Barsoom, at 43.963 miles, then the haad would be 2321.271 feet in length. As the earlier books are more consistent in describing the Martian units of time, I think that I will accept this earlier version of the Martian system of length measure as authoritative.
If one divides the haad into 200 parts, one obtains the ad, which would be 9.745296 feet; and dividing the ad into ten parts, one obtains the sofad, which would be 11.6943552 inches; and, finally, dividing the sofad into ten parts, one obtains the safad, which would then be 1.16943552 inches, or 2.9703662208 centimeters.
The FMJD dimensions for the squares of a 10 by 10 Checker board are from 3.5 centimeters to 4.5 centimeters; if we take 4/5ths of the dimensions provided by FIDE for the squares of an 8 by 8 Chess board, we get a range from 4 centimeters to 5.2 centimeters. Using the latter range as our primary standard, and attempting to convert it to a convenient multiple of a unit approximately 3 centimeters in length...
Until such time as the relevant Barsoomian authorities may be contacted directly, I shall humbly suggest that the regulation size for the squares of a tournament Jetan board should be about 1.5 safads (about 4.45555 cm, or 1 ¾ in.), with a permitted range from 1.35 safads to 1.7 safads.
On May 20, 1877, the Italian government passed a law aimed at standardizing the various units in use locally in different parts of the country, by defining them accurately in terms of the metric system.
Among the many units for which definitions were given were the units of length in use in Cremona:
Trabucco (6 Piede) 2.901 233 metres Piede (12 Once) 483.538 833 333 mm Oncia (12 Punti) 40.294 902 777 mm Punto (12 Atomi) 3.357 908 564 814 814 mm Atomo .279 825 713 734 567 901 234 567 901 234 567 mm
here expressed so as to make the repeating decimals visible, regardless of significance.
The different shoe sizes of Continental Europe and the English-speaking world may also be commented upon here.
The difference between one shoe size and the next in Britain and most of North America (excluding Mexico) is 1/3 of an inch. This unit actually is an obsolete named unit, the barleycorn. The difference between one shoe size and the next in Continental Europe (and, for that matter, in Iceland as well) is 2/3 of a centimetre. This unit is called the Paris point, and it may have originally come into existence because a quarter-inch is 6.35 mm - and a quarter of one-twelfth of the pre-revolutionary "pied du roi", a quarter of the old French inch, would be 6.76762 mm, even closer to 2/3 cm.
Also, an alternative scale of shoe sizes used in Europe, the Mondopoint scale, uses 5 millimetres as its fundamental unit.
Conventional European sizes are based on the length of the last (the form on which the shoe is made), and so an allowance for the foot having to be shorter than the last to fit into the shoe is required. Mondopoint sizes, on the other hand, are in terms of the length of the foot for which the shoe is intended.
North American sizes are also based on the length of the last, but with an offset.
Thus, the size of men's shoes is the number of barleycorns by which the length of the last exceeds eight inches.
A woman's shoe having the same numerical size is one and a half barleycorns shorter, or a woman's shoe made of the same length of last as another shoe for a man is one and a half sizes larger. So the size of a woman's shoe is the number of barleycorns by which the length of the last exceeds seven and a half inches, one and a half sizes corresponding to 1/3 inch plus 1/6 inch, which makes half an inch.
The difference between children's shoe sizes and men's shoe sizes is 12 1/3 sizes - so the size of a child's shoe is the number of barleycorns by which the length of the last exceeds 3 8/9 inches.
An inch being more than twice as long as a centimetre, being 2.54 centimetres, 1/3 of an inch is 1.27 times as long as 2/3 of a centimetre. This difference is enough that half sizes are common in North America and Britain, while generally uncommon in Continental Europe.
In Britain, adult shoe sizes are one less than men's shoe sizes in North America, and child shoe sizes are twelve greater than men's shoe sizes in North America; there are no separate men's and women's shoe sizes, and children's shoe sizes belong to the same scale of integer sizes as those of adults.