
Bonne's projection applies the true scale along the parallels of the Sinusoidal to the parallels of the Simple Conic instead of the parallels of the Plate Carrée, thus giving a projection that looks like this:

At one time, due to the simplicity of its construction, it was often used in atlases where equal-area maps were desired. However, it gives the least angular error along the standard meridian and the standard parallel, while falling off badly with distance from the center in other directions. Thus, Lambert's azimuthal equal-area projection is, quite reasonably, much preferred at the present time (although see the comments at the end of the previous page).
Thus, here is how a map of Eurasia might look on the Bonne projection:
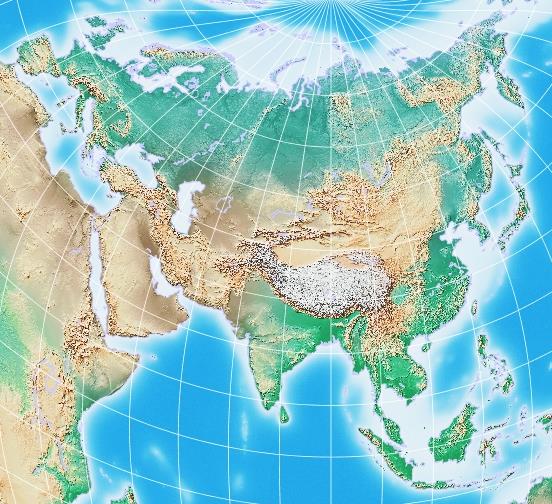
while a map of the same area, with the same center, on the Lambert Azimuthal Equal-Area projection would look like this:

It is interesting to note, however,
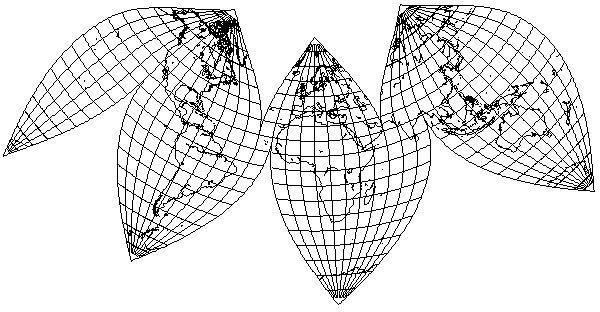
that Bonne's projection can be interrupted just as the Sinusoidal can be: this allows one to favor the Northern Hemisphere at least slightly in an interrupted world map, as shown above with a standard parallel of 20 degrees N.
One way to deal with having to interrupt a map, to avoid excessive distortion, at an awkward place, as was required in the Northern Hemisphere in the map above at 60 degrees East longitude, would be to draw part of the map twice.

This map places the Americas in the center, and the area from 60 degrees East longitude to 90 degrees East longitude, which includes India, is drawn twice, once on each side of the map, so that one can look on the West side and see India and Bangaladesh uninterrupted, or one can look on the East side and see Iran, Turkmenistan, and Kazakhstan uninterrupted.
Also, one can favor the Northern Hemisphere more strongly, as this map illustrates:
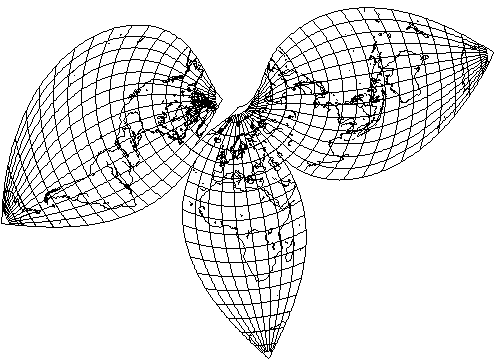
having a standard parallel of 50 degrees North latitude.
I think that this particular interrupted projection could be the basis for a very attractive map, along the lines of the illustration below:
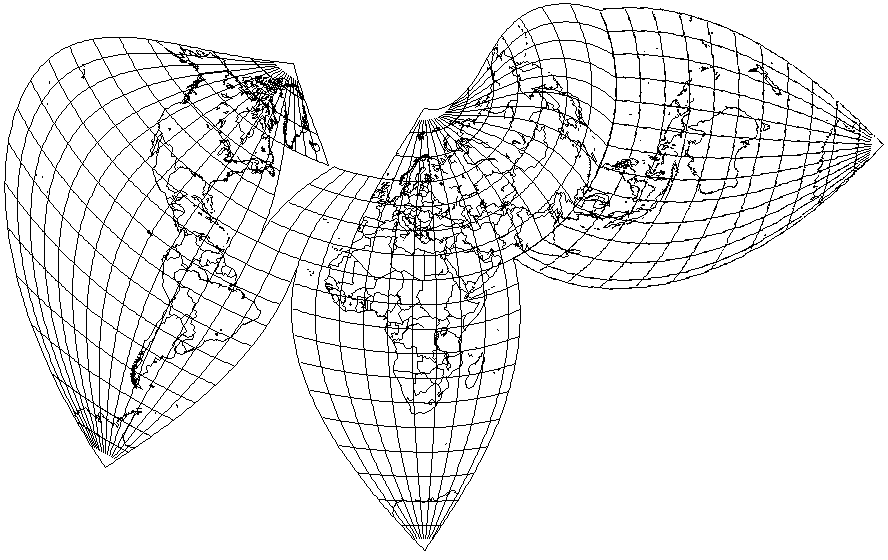
Here, the Americas, on the Western side of the map, are drawn with a standard parallel of 20 degrees North, but the standard parallel of 50 degrees North is used on the Eastern side of the map. This allows distortion for Asia to be kept relatively low.
But if the interruption to allow Australia and adjacent areas to also have low distortion, by being given a suitable standard meridian, is delayed until 20 degrees North latitude, so as to avoid interrupting Eurasia, that means that there is a kink in the map along 20 degrees North latitude. Steps can be taken to modify the projection to remove the kink, while the projection remains equal area, but at the moment they are too complicated for me to include in my map drawing program.
As well, since Bonne's projection provides uniform scale and conformality along its standard meridian, it is possible to mate the projection at the standard meridian with a Bonne's projection with a different standard parallel, or a Sinusoidal projection, or even the map Equator of a Sinusoidal projection in transverse aspect.
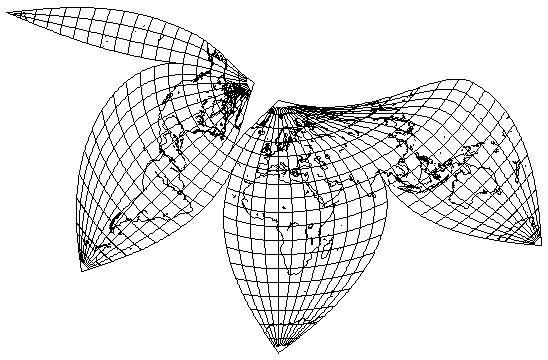
The image above illustrates a very simple example of this sort of thing; the left half of the map (from 170 degrees West to 10 degrees East longitude) has 40 degrees North as its standard parallel, while the right half has 20 degrees North as its standard parallel. Here, no interruption is made within the Eurasian landmass, as avoiding that would perhaps be the purpose of changing parallels like this, but the cost is severe distortion in Siberia. Achieving an equal-area world map with tolerable distortions everywhere, and yet no interruptions in major landmasses is difficult, but the flexibility of Bonne's projection together with the Sinusoidal projection lends itself to constructing patchwork projections of many kinds.
Here, as something of a geographic joke, is a map of Canada on an interrupted Bonne's projection, designed to minimize distortion in Canada's populated areas.

The standard parallel is 50 degreen North, just one degree north of the 49th parallel, the border between Canada and the United States in the western part of Canada.
North of that standard parallel, the standard meridian is 110 degrees West, so that it falls between Edmonton and Saskatoon, the only two major Canadian cities that lie significantly to the north of that standard parallel.
South of that standard parallel, the standard meridian is 76 degrees West, so that it falls between Toronto and Montreal, two large Canadian cities that are significantly to its south.
While this projection would not be considered for a general-purpose map of Canada, a map showing Canada's highways, railroad lines, and so on perhaps would be one for which this projection could be appropriate.
The following picture
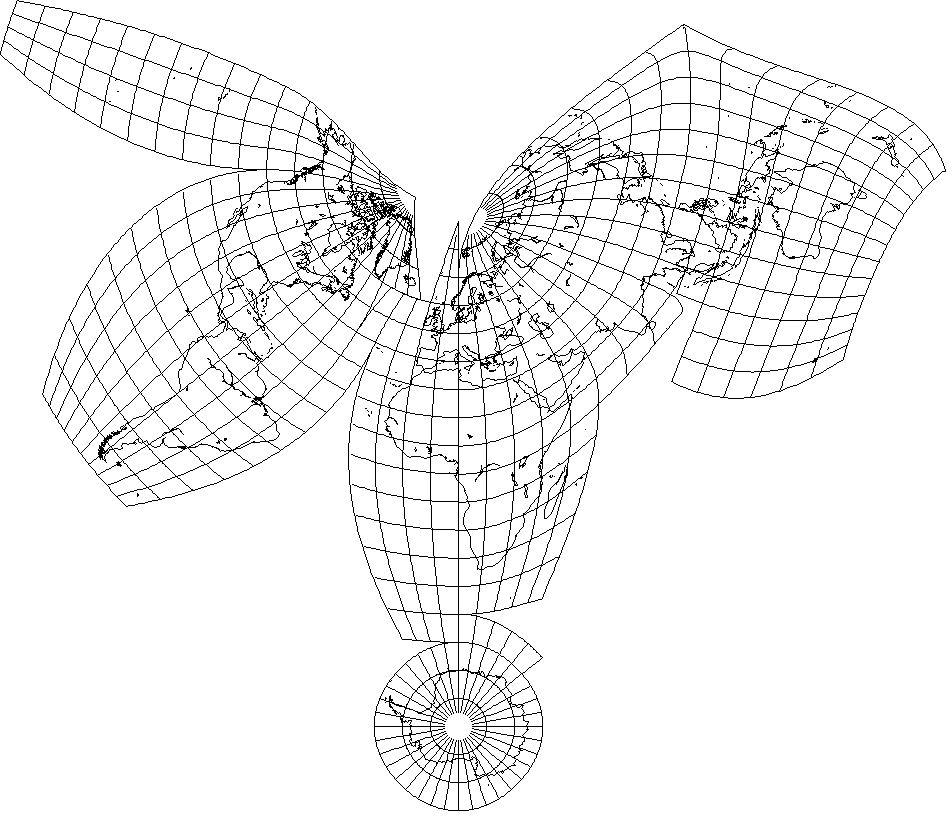
shows a world map which uses an interrupted Bonne's projection with 40 degrees North as standard parallel for most of the Western Hemisphere, and for the rest of the world, uses two transverse Sinusoidal projections (one of which is for Antarctica), one oblique Sinusoidal projection, and an oblique Bonne projection for Australia, whose standard parallel (in terms of the map construction) is 20 degrees. The difficulty of keeping the entire area from Africa to Australia connected has resulted in Africa being significantly distorted; Australia was severely distorted as well, before the second Bonne's projection was introduced. The boundary between the oblique Sinusoidal which is used for most of Asia and the transverse Sinusoidal used for much of Europe and Africa is a horizontal line pointing eastwards and starting from 10 degrees East longitude and 70 degrees North latitude.
Of course, Bonne's projection could also be mated, along a standard meridian, with the Polyconic, and the Sinusoidal could be joined, along the Equator, with the Mercator or the Miller Cylindrical; as long as the two projections are everywhere conformal and have uniform scale where joined, they do not have to have the same properties elsewhere.
One example of this is:
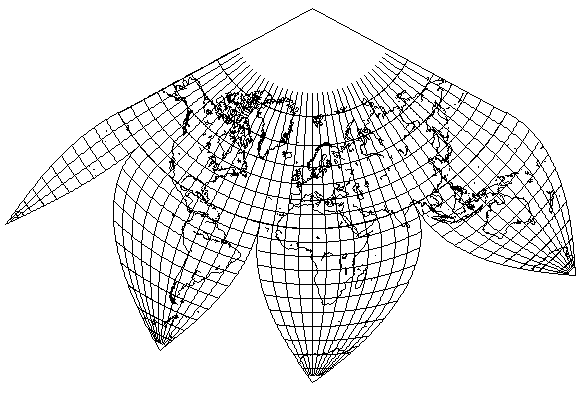
which uses a Lambert Conformal Conic north of the standard parallel for both projections of 20 degrees North, and Bonne's projection south of that parallel. A similar idea, but with more northerly standard parallels, is used in one form of Bartholomew's Regional Projection.
The actual Bartholomew's Regional Projection didn't use a Lambert Conformal Conic; instead, it used the Simple Conic, even though text associated with the map did use the word conformal - in a relative sense, of course.
Although I am, in general, hostile to projections with any sudden kink in them, such as the Goode Homolosine projection, in the case of the Bartholomew Regional projection, I feel constrained to make an exception, since it is a beautiful projection, and nothing else like it exists.
The story of the Bartholomew Regional Projection begins with the same basic projection under another name, the Kite projection. This appeared in Bartholomew's Citizen's Atlas in 1942, and looked like this:
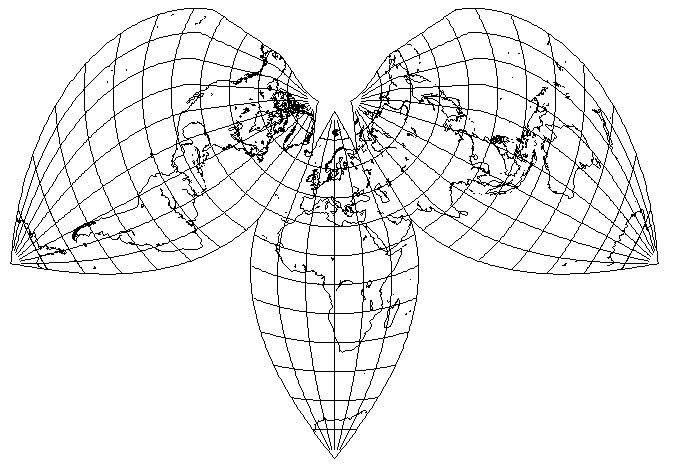
The map it was used on was a thematic map - indeed, all of the maps the projections of this family were used on were, to my knowledge, thematic; despite its high degree of fidelity, perhaps because of its inefficient use of space, no thought appears to have been given to ever using it for the primary political or physical map of the world in an atlas. But, in any case, because this one was used on a thematic map, specifically to illustrate the progress of political participation, the fact that the standard parallels for the areas with South America and with Australia were chosen more for symmetry than to reduce distortion in the land masses, may have been reasonable.
Note that, although there is a change in positioning by 10 degrees of longitude to avoid cutting land masses, the North polar area is interrupted in a similar fashion to the South part of the map. The standard parallels on all the maps of this family for the conic region, which are also its boundaries, are 22.5 degrees North and 66.5 degrees North, so that the North Temperate Area between the Tropic of Cancer and the Arctic Circle is on the conic projection.
Then came the Regional projection, which was first presented to the world in 1948 in the Edinburgh World Atlas of Advanced Geography. This is how it looked there:
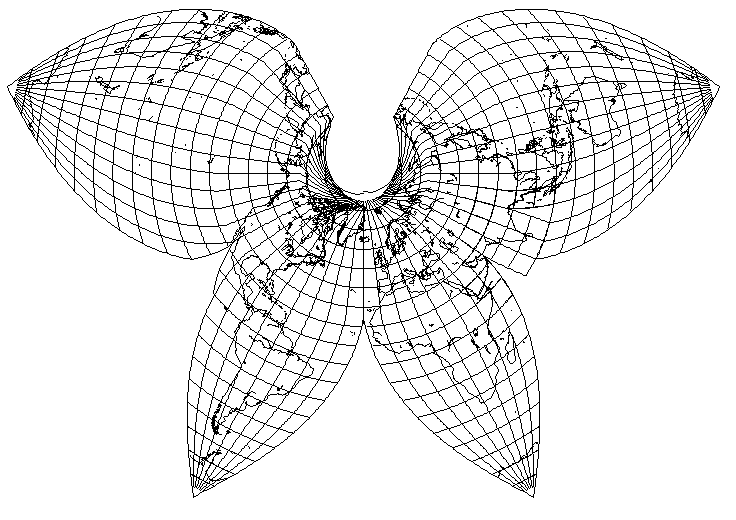
I could find no images of this map on the Web, and thus my original attempt to recreate it had one error; it did not show the diagonal line below Baja California to allow an additional 10 degrees of the Pacific Ocean to be grouped in the westernmost lobe.
This time, the interruptions of the North Polar Area found in the Kite projection were discarded as unnecessary, but given the large angular range of the projection, this led to considerable shear.
So that it is possible to present both an uninterrupted Australia with New Zealand beside it, and also most of the Pacific Ocean without interruption, an area 80 degrees in longitude was repeated twice.
And then in 1957, a modified version of the Bartholomew Regional Projection appeared in the Times Atlas of the World, a magnificent five-volume work. The version that appeared there looked like this:
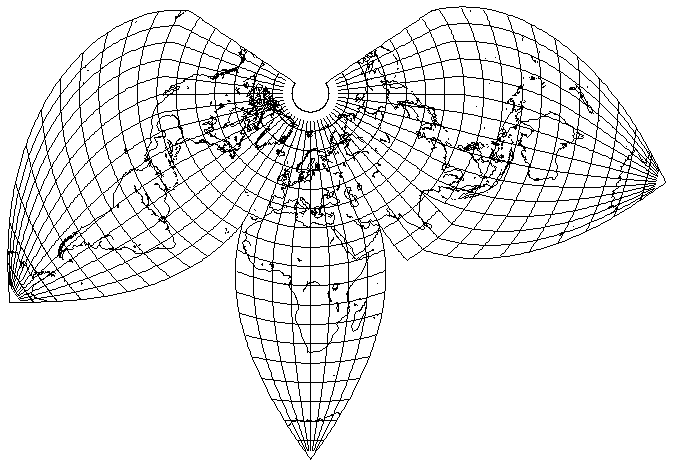
Now, the conic projection is extended to cover the North polar region, which I think is absolutely the right choice. However, on this map, but not its predecessors, the Bonne's projection was actually slightly modified where it joined the conic projection so as to eliminate the kink. Not only would it be difficult to see at this scale, but I don't know how it was done to duplicate it.
Personally, I would be inclined towards using slightly different parameters for the Regional projection, with the intent of making more general use of the result:
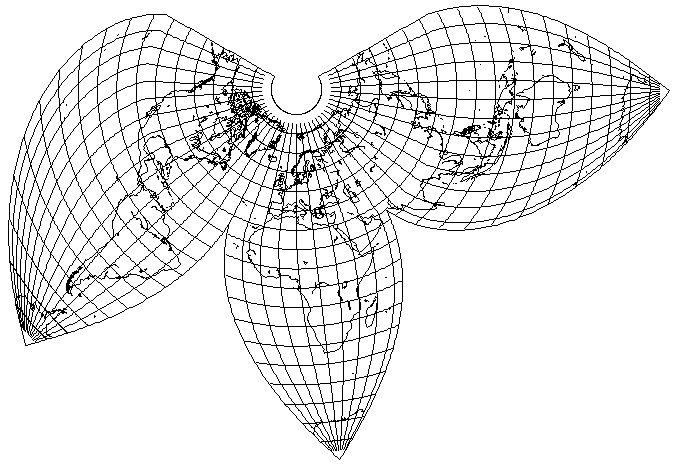
As in the version for the Times Atlas, it is conical to the pole.
The standard parallels are different, going from 25° N to 60° N. There seemed to be no particularly good reason for setting the standard parallels to the limits of the Temperate Zone specifically. The southern standard parallel is moved northwards slightly, because the continental shape of Asia allows for a slightly deeper interruption of the projection. The northern standard parallel is moved southwards, as the extreme Arctic areas are of limited interest, and it is better to reduce distortion elsewhere. And the standard meridian for Australia is improved, but Africa does not look as good, apparently due to the change in standard parallels.
One other "improvement" I've considered making to Bartholomew's Regional Projection is to use the conformal conic for the latitudes above the northern standard parallel. That way, the problems with the first two versions, and the extreme stretching of the third, are both avoided. However, there is a simpler way to do this. If interrupting the Arctic regions so that they are in three pieces is excessive, and keeping them uninterrupted for the whole globe is inadequate... why not only use two lobes of Bonne's for the Arctic regions?
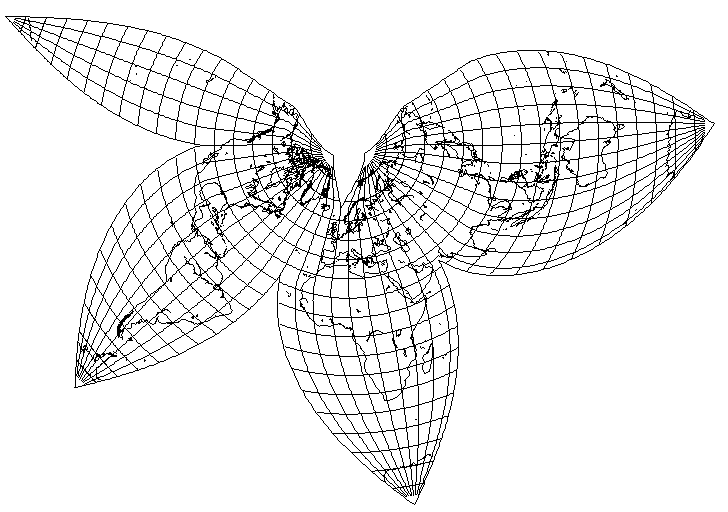
The extra lobe in the south, sticking out an additional distance to the west, so that at least some of the islands of the South Pacific might stand more closely in their correct relationships to each other is probably excessive, but otherwise I think this is an eminently suitable application of Bartholomew's Regional Projection.
Retaining the equal-area property, one can mix a transverse Cylindrical Equal-Area projection with an oblique Bonne's projection having a standard parallel of 10 degrees in the map coordinate system, and centered on 110 degrees East longitude and 80 degrees North latitude, and another Bonne's projection with standard meridian 110 degrees East longitude and standard parallel 10 degrees South latitude as follows:

This projection keeps the shapes of the major land masses reasonably accurate, and also avoids interrupting the area in the North Atlantic and around the Arctic Circle that shows the relationship between North America and Eurasia.
For ease of understanding, the standard parallels are shown in orange, and the regions in which the three projections are used each have their own color.
It can be seen that the area in which the Cylindrical Equal-Area projection is used is too wide for it to be replaced there by the Sinusoidal projection. However, an equal-area projection derived by placing the world on a Cylindrical Equal-Area projection on one scale on a Cylindrical Equal-Area projection at a larger scale, to give a projection with a flat pole-line and sinusoids for meridians could be used; the Eckert VI projection and the MacBryde-Thomas Flat-Polar Sinusoidal projection are projections of this type; however, it is necessary that the equator be the standard meridian of the projection. One could even use Adams' Equal-Area projection at some points, and if one wished, one could put half of Antarctica on Lambert's Azimuthal Equal-Area projection with its help.
The Bonne projection by itself seems to do so well, however, that I keep wanting to keep things simple; yet, no matter what I do, I can't avoid having to interrupt Asia to avoid excessive distortion. Still, if that can be accepted, one can get results like:
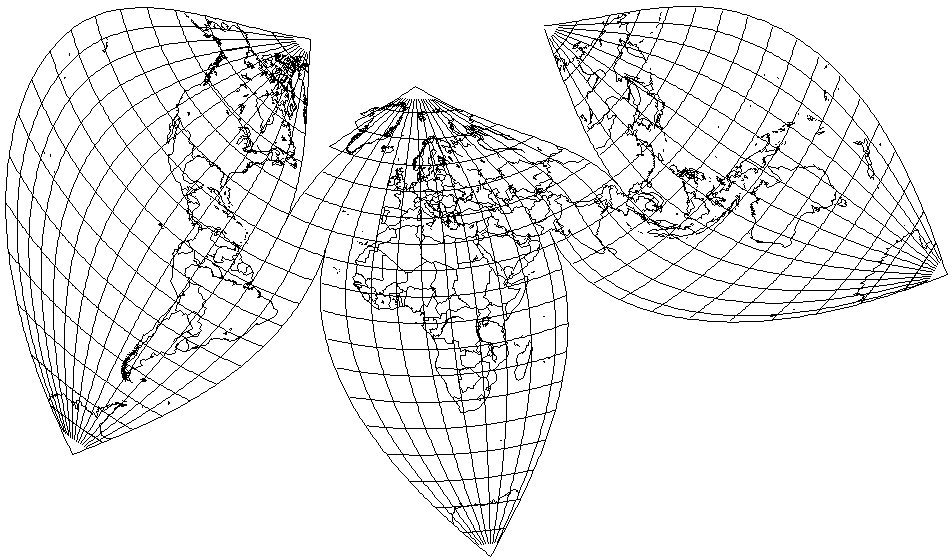
where I pushed the standard parallel as high as possible, to 25 degrees North, and then at first the standard meridian for Europe as far East as I could, to 25 degrees East, but I still found that distortion was just too high for eastern Asia, and an interruption was needed, so I interrupted Russia in the Siberian wastes, Mongolia, and the interior of China, and then chose an ideal standard meridian for each of the two parts.
Another way to avoid having to interrupt Asia would be to attempt an interrupted oblique Bonne's projection. Here is one of my recent attempts:
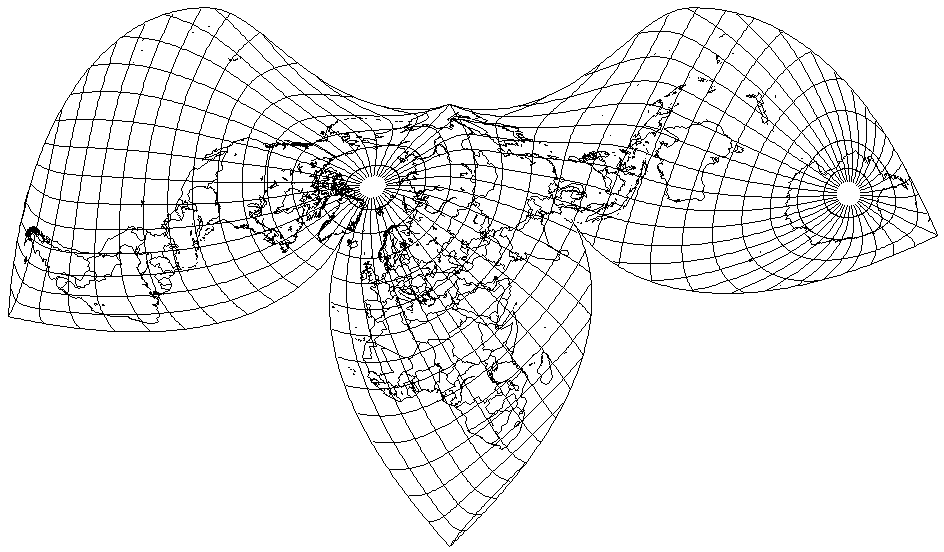
It comes close to what I'm looking for, but I'm not entirely happy with how South America turns out, and the distortion to which Japan is subjected is simply not acceptable. But when I try to improve one of the problem areas, I end up making things worse somewhere else.
Still, I found one combination that is an improvement for the most part,
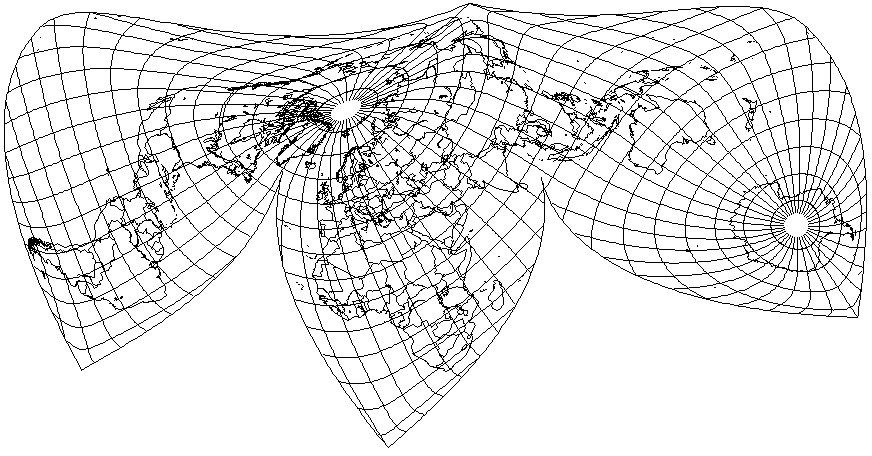
despite the fact that I had to change the standard parallel to 15 degrees from 30 degrees. Japan and South America are much improved. Unfortunately, North America is significantly worse off, although things only get really bad around Alaska and the Yukon.
Since the standard parallel has been moved so much closer to the Equator of the projection, the obvious solution is to interrupt the Northern portion of the projection.
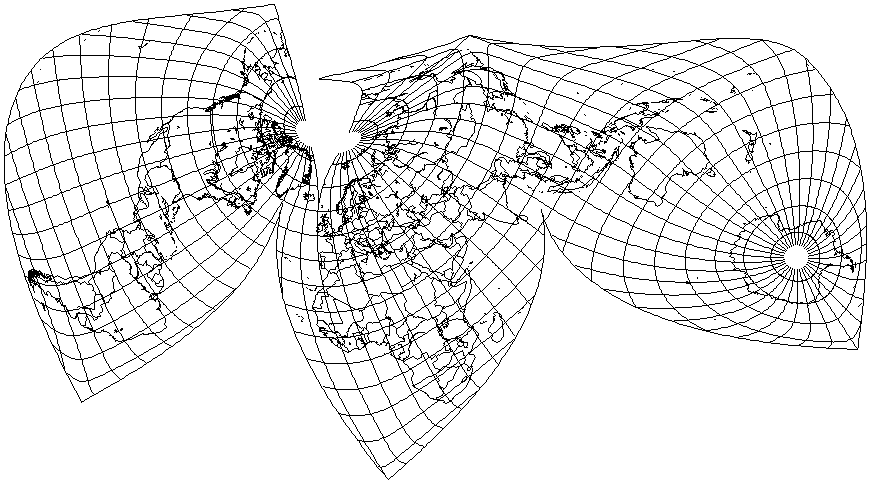
Doing so completely solves the difficulties with North America. The opportunity has been taken to redraw, with lower distortion, a portion of Eastern Siberia close to Alaska, while leaving in the original version that is attached to the body of Asia.
As for South America, since 100 degrees West in projection coordinates is a standard meridian in both the northern and southern parts of the projection, changing the standard parallel to the west of it from 15 degrees north to 50 degrees south (again, in projection coordinates) solves that issue, as the diagram below illustrates:
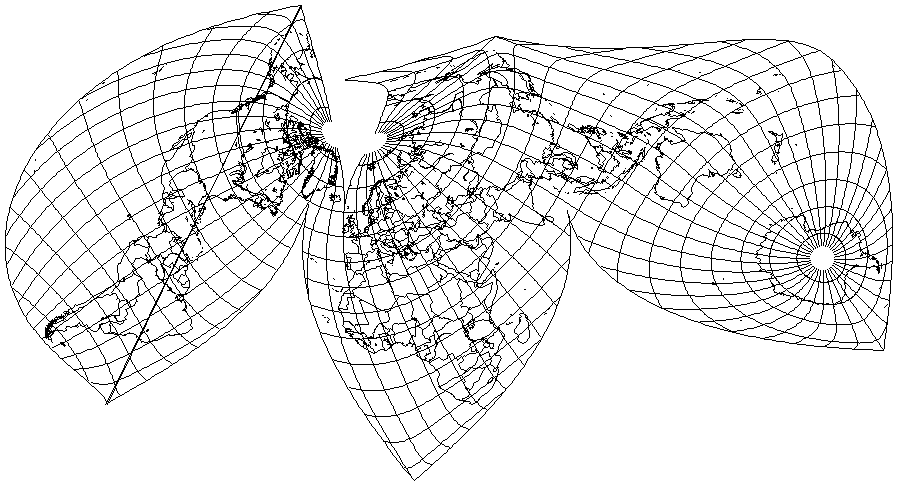
Can something be done for Japan and Korea? Changing the standard parallel from 15 degrees north to 65 degrees north, in projection coordinates, does indeed improve their appearance, but because the standard meridian in the northern part of the map is 30 degrees east in projection coordinates, doing so means that an interruption needs to intrude into Manchuria:
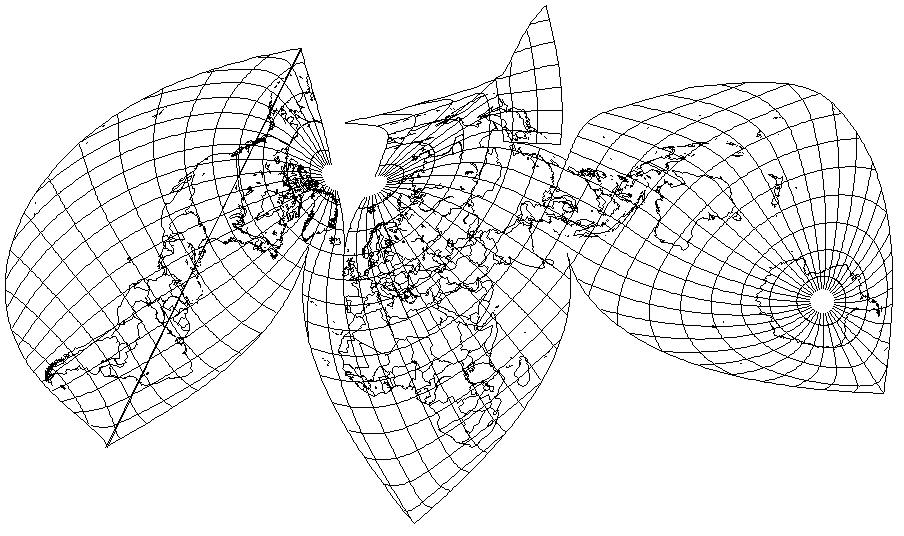
Perhaps it is possible to modify the projection enough to avoid this without causing serious problems elsewhere, by moving the standard meridian in that area.
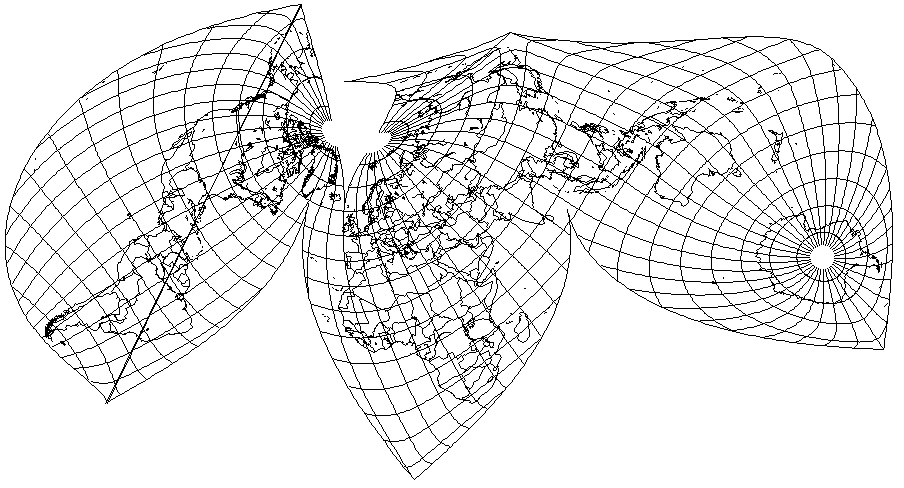
Instead, it seems that moving the standard meridian to make taking this step easier has also improved the appearance of Japan and Korea enough so that the effort is not needed.
None the less, it still can be done:

and while there is only a slight reduction in shear, there is still a significant reduction in stretching, so it is useful to do this.