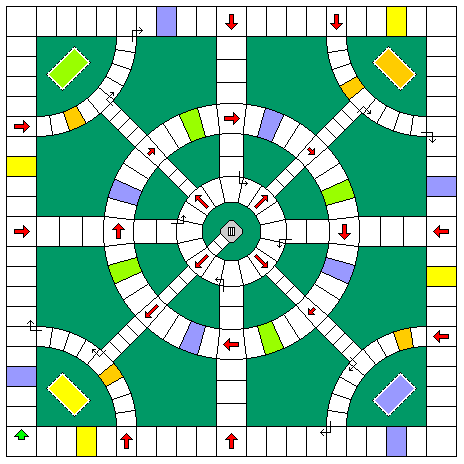
Speaking of more complicated kinds of games, back when I was a wee lad, I had always dreamed of finding a board game that was just a bit more fascinating and engrossing than those typically available. Something like this:

The way movement takes place in the game, to the extent that this is not obvious from the board, is as follows:
On the outer periphery of the board, movement is clockwise, and players throw two dice to determine the number of spaces to advance on each turn. This also applies to the large circle inside the board.
There are eight arrowed spaces on the outer periphery of the board that lead the player inside the board. When a player lands on one of these, on the next turn, he uses only one die to advance in the direction of the arrow.
Four of those arrowed spaces lead players on short side trips, on curved paths that avoid the four corners on the board. Note that one of these paths skips the square with a light green arrow in the lower left corner of the board as shown; this is the square which grants players their normal salary when they pass it, and this salary is missed when it is bypassed with the side trip. The side trip paths are circular, and note that movement on them, although clockwise relative to the center of the board as a whole, is counter-clockwise relative to the centers of the circles of which those paths would form a part.
Arrow squares inside the board also change the direction of a player's movement as well. The ones that cause the player to move to the large circle also lead to using two dice for each move, instead of one die, once more.
When a player lands on one of the four arrowed spaces in the middle of one of the sides of the outer periphery of the board, the normal course of events is that the player will advance to the small circle in the center of the board, there to remain for a short time until landing on one of the four arrowed squares in that circle, and then to proceed on a diagonal path which then brings the player to the second half of one of the four side trips in the corners of the board.
However, on the way in, the player has one chance to land on an arrowed square by means of which he can enter the large circle within the board. As well, on three of the four diagonal paths heading outwards, the player has one arrowed square which forms a second chance to enter the large circle. On the fourth one, the arrow points along the diagonal path instead, and that arrowed square is the one by which players moving along the large circle are returned to the outer periphery of the board.
Note that there are four stacks of cards that bring surprise events when landing on the colored squares which cause a player to draw one of those cards. Squares for drawing the blue cards are present both on the outer periphery of the board and on the large circle within the board. The yellow cards belong to the outer periphery of the board only, and the green cards belong to the large circle within the board only. The orange cards are available only within the first half of the four side trips on the board.
It is intended that the outer periphery of the board is the main play area where players start building their fortunes. The large circle is an attractive place to be, where players can earn money more quickly, but the stakes are also higher there, and it is generally only advantageous to be there once a player has already accumulated more money than he started with in the other parts of the board. Note that this circle does not contain a salary square which provides the player with a certain amount of money when it is passed. The side trips in the corner provide players with the chance to accumulate valuable prizes, including the orange cards, many of which, perhaps about a third, are kept after being drawn, and allow the player to choose his next move instead of rolling the dice.
The problem with a game structured that way, of course, is that, particularly when there are two players, the game would threaten to become less interesting and unbalanced while one player is on the outer periphery and the other is in the large circle within the board.
Note that when a player is sent to jail, the jail being located in the very center of the board, he exits by the one diagonal path that doesn't provide the chance to start moving along the large circle within the board, and also ends up on the side trip path whose exit is the farthest one from the annual salary square.
Or similar principles might be applied to a somewhat more elaborate game board:
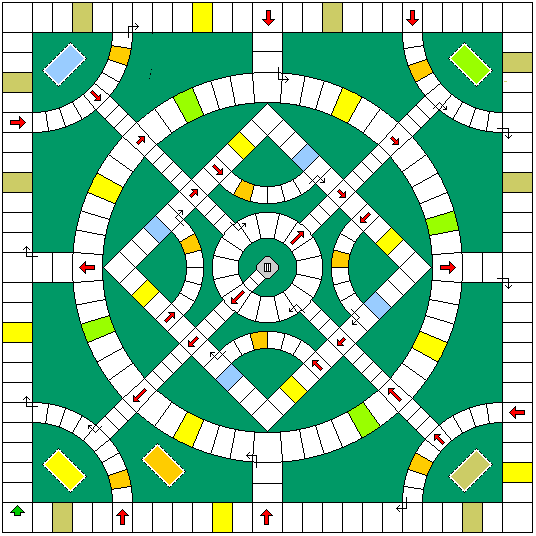
Here, the striking diagonal square is relatively difficult to reach while the large circle within the board is only moderately difficult to enter. The central circle performs the same function as before, but this time with two entrances and two exits instead of four entrances and four exits.
Two squares on the main board lead to entering the large circle, and four lead to entering corner paths.
On two of the four corner paths, and on one space on the large circle, are squares which set the player on the two paths to the center circle. On these paths, there is the opportunity to enter the diagonal square in the center.
There are two paths back out from the center circle; one of these gives a second chance to enter the diagonal square; the other one, which is the road a player takes when leaving jail, does not. That path allows no side turnings, and is instead the path by which a player would leave the diagonal square, and one of the paths by which a player would leave the large circle. It leads into the corner path through which a player would miss the salary square on the main board.
Finally, to go even further, perhaps one might design a hexagonal game board with the following structure:
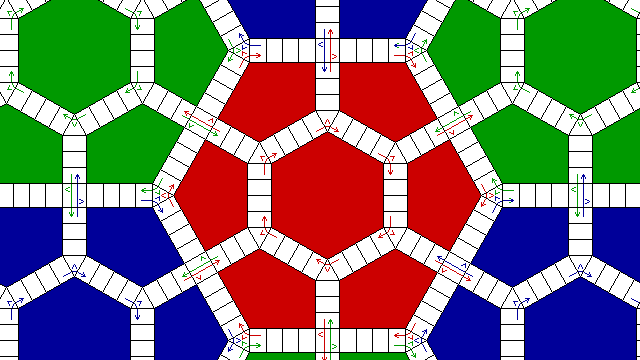
One red hexagon surrounded by three blue and three green ones takes this principle to a large enough scale. Movement within each hexagon is clockwise, as the arrows at the various types of intersection squares or triangles indicates.
Many different games based on different principles have been devised for play with a theme based on stock market trading. One of the reasons for this is that none of the existing principles is fully satisfactory.
Two of the principles that have been used in such games, I feel, could be combined to make a more realistic stock market game. But the problem with that is that the result is, I fear, too complicated.
A set of tracks, giving the value of each of, say, six stocks might have to the left of it a table like the following:
2 3 4 5 6 7 8 ---------------------------- 100 144 192 240 288 336 384 [SPLIT] 92 138 184 230 276 322 368 (slow) 88 132 176 220 264 308 352 [SPLIT] 84 126 168 210 252 294 336 (slow) 80 120 160 200 240 280 320 [SPLIT] 76 114 152 190 228 266 304 ... 44 66 88 110 132 154 176 40 60 80 100 120 140 160 36 54 72 90 108 126 144 ... 20 30 40 50 60 70 80 16 24 32 40 48 56 64 12 18 24 30 36 42 48 (slow) 8 12 16 20 24 28 32 (slow) 4 6 8 10 12 14 16 (slow)
Given some arrangement of dice that selects a stock to move up or down, and indicates that it will move up or down 1, 2, 3, or 4 steps, indicating some lines on the scale as (slow), meaning that stocks move up or down only one step, instead of the number of steps indicated, when starting from those lines, means that having one end of the scale where stocks become worthless, and another end where, to keep the length of the scale reasonable, each share is replaced by two shares of half the value, no longer means that there are situations where a stock can go down only one step, or up only one step, but several steps in the other direction.
Making the vertical scale a completely dead-even proposition, however, is not in itself an improvement.
But adding the horizontal scale now lends a significant dimension of skill to the game.
The number heading each column of numbers is a multiplier.
The multiplier is limited to the fixed range of values from 2 to 8, and can't go off the ends of that range. 5 would represent normal economic times, 3 would represent a bust, and 7 would represent a boom.
In addition to causing the values of all the stocks to change suddenly, because the range is strictly limited, all the values of the multiplier are not equal. While motion of an individual stock vertically is inherently even-handed, with a stock, whether it is high or low, still being equally liable to move up or down, if the multiplier is low, someday it can be expected to move up; if the multiplier is high, someday it can be expected to move down.
So it would be trivially obvious to always buy stocks when the multiplier is low, and always sell when the multiplier is high.
If one balances that with making stocks less likely to move up, and more likely to move down, when the multiplier is low, and more likely to move up, and less likely to move down, when it is high, and also making dividends more likely when the multiplier is high, then two conflicting factors are present.
The balance between them would depend on how frequently the multiplier changes, compared to the greater frequency of vertical movement (presumably, one stock would move vertically, or pay a dividend, on every, or nearly every, turn). This could vary during a game, depending, for example, on how many cards calling for a multiplier change are drawn from a deck of cards calling for random events, or whether any players on a gameboard are near a space that calls for a multiplier change.
The vertical motion gives individual stocks individual changing values, and it can be controlled so as to counterbalance the horizontal motion of the multiplier. This would afford the opportunity for subtle and complex strategies to improve one's chance to profit.
As I've noted, I was thinking in terms of this stock market as being part of a more conventional game, in which players move their pieces around a track, and on some spaces, draw cards telling them to win or pay money and move ahead or back and so on. If the board is large enough, players might roll three dice to move.
If so, even with 6 different stocks (let's just call them A, B, C, D, E, and F) if each roll of the dice was looked up on a chart like that above, perhaps before the total is also used by the player to move, there would be enough possibilities to offer a range of vertical movement:
111 - A div 5 112 - A -3 113 - A -2 114 - A div 2 115 - A +2 116 - A +3
222 - B div 5 122 - B -3 223 - B -2 224 - B div 2 225 - B +2 226 - B +3
333 - C div 5 133 - C -3 233 - C -2 334 - C div 2 335 - C +2 336 - C +3
444 - D div 5 144 - D -3 244 - D -2 344 - D div 2 445 - D +2 446 - D +3
555 - E div 5 155 - E -3 255 - E -2 355 - E div 2 455 - E +2 556 - E +3
666 - F div 5 166 - F -3 266 - F -2 366 - F div 2 466 - F +2 566 - F +3
124 - A -1 234 - A div 1 125 - A +1
134 - B -1 126 - B div 1 135 - B +1
235 - C -1 136 - C div 1 145 - C +1
245 - D -1 146 - D div 1 236 - D +1
346 - E -1 156 - E div 1 246 - E +1
356 - F -1 345 - F div 1 256 - F +1
with the two remaining possibilities, 123 and 456, calling for changes of the multiplier; 123 might call for a new multiplier to be chosen at random, while 456 would call for its value to take one step up or down.
One way a random change in the multiplier might be achieved is to have, somewhere inside the main area of the game board, a small circular track with different multiplier numbers on it, with a piece that would be advanced by the roll of dice when a random change is desired.
The multiplier step change would involve rolling a single die, reducing the multiplier by 1 if 1, 2, or 3 is rolled, and increasing it by 1 if 4, 5, or 6 is rolled, and advancing the piece to the next space with that number on it.
The distribution of numbers on the track should be somewhat like a normal distribution. For example, there might be 36 numbers along the track, in the following distribution:
2) 3 3) 4 4) 7 5) 8 6) 7 7) 4 8) 3
perhaps in the following sequence:
2 6 5 3 6 4 8 5 6 7 5 4 2 6 3 5 7 4 8 6 5 3 4 5 2 6 4 7 5 4 8 4 3 5 7 6
But this would seem to be certainly far too complicated, requiring players to look up the meaning of a roll of three dice at every move.
A simplification would be to have players, when rolling three dice to move, also roll one special die to indicate the stock to be affected. Then, at least when the multiplier is at 5, the significance of the total of the three dice would be:
3 div 5 11 up 1 4 div 2 12 up 2 5 multiplier random 13 up 3 6 div 1 14 up 4 7 down 4 15 div 1 8 down 3 16 multiplier step 9 down 2 17 div 2 10 down 1 18 div 5
Some additional complications, however, would be nice for adding to the realism of the stock market simulation, even though what has been described already seems to be beyond the practical level of complication available in a board game. (And, what is worse, hiding the complication by using a computer would mean that the multiplier and the vertical position of a stock wouldn't be visible separately, thus preventing this scheme from having its benefit of permitting more sophisticated strategy; thus this obvious option for dealing with complexity seems to be at least limited in its applicability.)
The first such complication would be to reduce the predictablity of the multiplier's return to its other possible values by requiring three dice to be rolled after every change of the multiplier, to indicate stocks the value of which is unaffected, or nearly so, by the change in the value of the multiplier.
Each number from 1 to 6 on the die would correspond to one of the six stocks. If all three dice roll the same number, only one stock is unaffected; if all three dice roll different numbers, all three stocks indicated are unaffected. When two dice show the same number, and the remaining die shows a different number, the stock indicated by the two dice is unaffected, and the stock indicated by the remaining die has the effect of the change of multiplier reduced to half its value.
This is achieved through vertical movement of the stock, which follows the change of the multiplier, but which is part of a single move, and takes place before any further stock trading is allowed.
This movement is to the vertical position that would give the stock the desired value under the new multiplier, subject to certain limitations.
The desired value is either the value the stock had in its present vertical position under the old multiplier, for a stock to be unaffected, or the average of that value and the value the stock would have in its present vertical position under the new multiplier in the case where the effect of the multiplier change is to be reduced by half.
The three limitations are:
To illustrate the effect of the third limitation, let us suppose that a stock has the value of $112 per share under the existing multiplier of 7, and the multiplier has now changed to 3, and this stock is to be unaffected in value by the multiplier shift.
Under the new multiplier of 3, the two available values nearest the desired value of $112 are $114 per share and $108 per share. Although $114 per share is only $2 away from $112, and $108 per share is $4 away from $112, the stock is moved upwards vertically to the position that will give it a value of $108 per share under the multiplier of 3 since this compensation is intended to eliminate if possible, or reduce, the downwards effect of a decrease in the multiplier, not create an increase in the stock's value.
Similarly, if the multiplier increases, and a stock is unaffected, if the stock's value cannot be completely unaffected, it may increase slightly, but it may never decrease at all as a result of the vertical motion downwards to eliminate or reduce the upwards effect of the increase of the multiplier.
If a stock had a value of $112 per share under the existing multiplier of 7, and the multiplier changed to 3, and it was to experience half the effect of the multiplier shift, then, since its value if it had not been moved vertically under the new multiplier would be $48 per share, the desired value is, in that case, $80, which is halfway between $112 and $48.
Even where the effect is halved instead of eliminated as far as possible, the rule that only undercompensation and not overcompensation is allowed still applies. Under the multiplier of 3, the available values near $80 are $84 and $78, and so the stock is moved vertically upwards to have the value of $78 per share under the new multiplier of 3.
The second additional complication would be to allow it to be visible that some stocks are likely to perform better than others. One could have a small board where each of the 6 stocks could be either normal, a star performer, or a poor performer. The multiplier would be the same for all stocks, but rolls of the dice indicating vertical movement would be interpreted as for a multiplier of one greater (if possible) for a star performer, or one less (again, if possible) for a poor performer.
Such a status for a stock would be temporary. Again assuming the stock market element in the game is accompanied by a conventional board game, one way to achieve that is to have all the stocks be reset to normal performance whenever a player lands on or passes the start square on the board (which might stand for a monthly payday when one's salary is recieved).
Another factor not yet dealt with is that dividends should be proportional to a stock's value.
This might work as follows:
This could be indicated through color coding in the chart of values for each horizontal line in the vertical movement chart for each multiplier.
On the other hand, a game that involves players landing on properties owned by other players and paying rent on them - like Monopoly... or The Landlord's Game or Finance or Easy Money - should not have a complicated board in which players can spend long periods of time immune to landing on the other players' properties.
Instead,
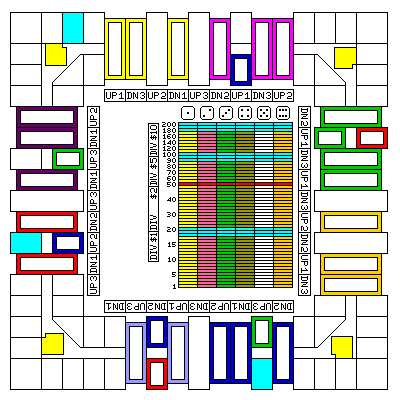
perhaps something like this might be a suitable board for a more elaborate game of this genre.
Players move around the outer board until they reach a certain level of wealth. They pay one-tenth of the "normal" rent on properties when on the outer board. The inner board doesn't have a space on the corner providing a monthly wage when it is passed.
As for stocks, a layout similar to the board for the game Stock Ticker is provided. But the value of a share of stock doesn't increase by uniform steps; instead, the step size is adjusted at intervals to be roughly proportional to the value of a share:
Share Value Price Increment $1 - $19 $1 $20 - $48 $2 $50 - $95 $5 $100 - $200 $10
To allow the values of stocks to change during the game without intruding into gameplay, when players roll two dice to move, the two dice indicate the two stocks that change value in the same fashion. A stock may move up or down 1, 2, or 3 steps; this depends on the square the player lands on; if the player lands on a square near the corners, no change in stock values takes place.
The change, up or down, and 1, 2, or 3 steps, is indicated by the writing in the strips above the spaces on the board, towards the center of the board. If doubles are rolled, then the one stock indicated by the number on both dice pays a dividend instead, of 1, 2, or 3 units: the size of a dividend unit is given by the legend to the size of the track of stocks. A stock valued at under $10 does not pay dividends.
Stock changes and dividends would be the first thing to happen in a turn, immediately after a player rolls the dice and moves his man, before any other actions are taken in the turn, so that no one can buy or sell stocks at their old value when their new value is already known.
When a stock hits $180, $190, or $200 in value, a stock split takes place; either twofold, to $90, $95, or $100 respectively, or tenfold, to $18, $19, or $20. The rule might be that the first stock split is twofold, and any subsequent ones are tenfold to keep splits from happening too often.
Both the possibility of dividends, and the increasing step size on the scale of share values, which means that stocks having certain values will move up by larger amounts than they would move down, makes holding stocks profitable, and, thus, represents a source of productivity in the game.
Note that on the four sides of the board, the first side is biased towards stocks moving up, the second and fourth are evenly balanced, and the third side has a bias towards stocks moving down which balances the bias of the first side. This allows players to exercise some degree of skill in anticipating a favorable or unfavorable climate for stocks, but probably not on a practical time scale. One way that might work would be to have some of the random event cards drawn by players set global rules such as changing "up 3" to "up 2" during an unfavorable time, and changing "down 3" to "down 2" instead during a boom. A more severe economic setback might be represented by a more elaborate rule, such as changing both "up 3" and "up 2" to "up 1", but only for stocks with values of $50 or greater, so that stocks aren't driven too quickly off the board.
The problem in the design of a game like this might be to ensure that both stocks and properties play a significant role. Properties are the means to directly address the primary goal of bankrupting the other players to win; stocks appear to merely be a way to earn a little extra money with one's spare cash. But that might be enough.
Monopoly, Finance, Rich Uncle, Stock Exchange, and even The Landlord's Game are trademarks of Hasbro as it currently owns Parker Brothers; Easy Money is also a trademark of Hasbro as it currently owns Milton-Bradley. Stock Ticker is a trademark of Copp Clark.
The Landlord's Game is currently a registered trademark of yourPlay LLC, owned by Thomas Forsyth, who made and sold a modern reproduction of the 1906 game. His original reproduction of the 1906 game was, however, titled Progress and Poverty; apparently, it is only afterwards that he acquired the rights to the original name. According to his web site, at least as I understood what I read there, the trademark lapsed when Hasbro purchased Parker Brothers because while Parker Brothers had a de facto or common-law trademark to the name The Landlord's Game from having published a version of that game, it had never registered its trademark; thus, as Hasbro didn't also publish this game, Parker Brothers only having published it very briefly, it became available.