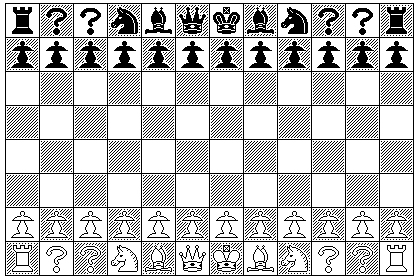
The game described here attempts to retain a rectangular form for the board, while still preserving the ability to place the King in an intact Castled position similar to that possible in normal chess.
To that end, while the first of three basic starting points in constructing variants in this game is the same as that used for Random Variant Chess:

it leads to only fourteen possible variants:
1) Giraffe, Camel, Camel, Giraffe 2) Zebra, Camel, Camel, Zebra 3) Princess, Camel, Camel, Empress 4) Princess, Giraffe, Giraffe, Empress 5) Princess, Zebra, Zebra, Empress 6) Camel Princess, Camel, Camel, Camel Empress 7) Camel Princess, Giraffe, Giraffe, Camel Empress 8) Camel Princess, Zebra, Zebra, Camel Empress 9) Giraffe Princess, Camel, Camel, Giraffe Empress 10) Giraffe Princess, Giraffe, Giraffe, Giraffe Empress 11) Giraffe Princess, Zebra, Zebra, Giraffe, Empress 12) Zebra Princess, Camel, Camel, Zebra Empress 13) Zebra Princess, Giraffe, Giraffe, Zebra Empress 14) Zebra Princess, Zebra, Zebra, Zebra Empress
Just as the Princess has the move of a Knight or a Bishop, the Camel Princess has the move of a Camel or a Bishop, the Giraffe Princess has the move of a Giraffe or a Bishop, and the Zebra Princess has the move of a Zebra or a Bishop. Note that the Camel Princess, like a Bishop and like the Camel, is confined to squares of only one color, and is thus weaker than the others.
Similarly, as the Empress has the move of a Knight or a Rook, the Camel Empress has the move of a Camel or a Rook, the Giraffe Empress has the move of a Giraffe or a Rook, and the Zebra Empress has the move of a Zebra or a Rook. As the Rook can travel to squares of both colors, the Camel Empress is not weaker than the other members of the Empress family.
When this layout is the starting point, the King Castles by moving four spaces, when Castling either to Kingside or Queenside, and the Rook then jumps over the King to the next space beyond.
The second basic starting point allows new pieces to be brought in that don't have the power of leaping over the row of Pawns in the front by placing the new pieces between the King and the Queen:
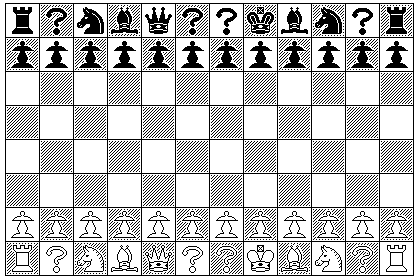
When this layout is the starting point, the King Castles by moving three spaces, when Castling either to Kingside or Queenside, and the Rook then jumps over the King to the next space beyond.
Thus, King's-side Castling is still preserved, while the disadvantage of Queen's-side Castling increases.
Alternatively, the Castling move can be left at four spaces, and the integrity of Castling on both sides can be maintained, by changing this second starting point to this instead:
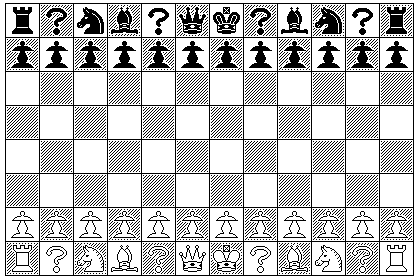
With either of these starting arrangements, the number of possible variants is considerably increased. The possibilities for the outer two pieces are:
15) Camel, ?, ?, Camel 24) Giraffe, ?, ?, Giraffe 33) Zebra, ?, ?, Zebra 42) Princess, ?, ?, Empress 51) Camel Princess, ?, ?, Camel Empress 60) Giraffe Princess, ?, ?, Giraffe Empress 69) Zebra Princess, ?, ?, Zebra Empress
which are then combined with the possibilities for the inner two pieces to create a variant:
0) ?, Tiger, Cannon, ? 1) ?, Man, Walker, ? 2) ?, Wazir, Fers, ? 3) ?, Rhinoceros, Griffin, ? 4) ?, Cannon, Leo, ? 5) ?, Duke, Count, ? 6) ?, Counsellor, Squirrel, ? 7) ?, Counsellor, Star, ? 8) ?, Archer, Sprinter, ?
and, thus, as the first starting arrangement leads to fourteen variants, variants 1 through 14, this second starting arrangement leads to sixty-three additional variants, variants 15 through 77.
Similarly, the third starting arrangement can either be:
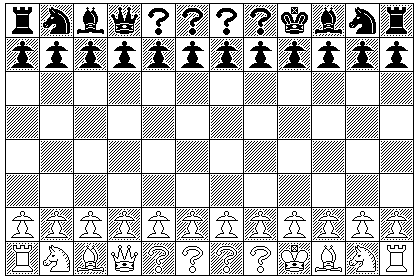
which would cause Castling to now begin with a two-square move of the King, as in normal Chess, or this improved form:
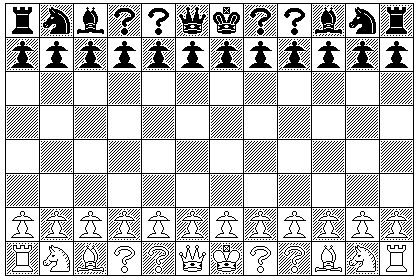
where Castling again remains a four-square move of the King, and Queen's-side Castling is only at a one-square disadvantage.
An assortment of variants can be obtained by choosing the outer two pieces subject to change as follows:
77) Tiger, ?, ?, Cannon 81) Cannon, ?, ?, Leo 85) Counsellor, ?, ?, Squirrel 89) Counsellor, ?, ?, Star 93) Archer, ?, ?, Sprinter
and the inner two as follows:
0) ?, Man, Walker, ? 1) ?, Wazir, Fers, ? 2) ?, Rhinoceros, Griffin, ? 3) ?, Duke, Count, ?
leading to twenty more variants, for a total of ninety-seven.
As this is a limited list of variants, to increase their number without disturbing Castling, what remains is to substitute something for the Queen.
The possibilities are:
1) Amazon 2) Griffin 3) Empress 4) Camel Empress 5) Giraffe Empress 6) Zebra Empress 7) Rhinoceros
with the following special rules:
This avoids duplicate combinations and combinations with unpaired duplicate pieces, and it increases the number of combinations from 97 up to 679, which should be adequate to eliminate prepared opening variations.
A further increase in the number of combinations is easily possible, however.
The basic 97 combinations are divided into three groups; fourteen with two additional pieces that must be able to leap over the front row of Pawns beyond the Knight, sixty-three with one additional piece with this property beyond the Knight, and twenty with no such piece beyond the Knight.
In those last 20 combinations, the Knights can be replaced by Camels, Giraffes, or Zebras, increasing the number of combinations of that type to 80.
Thus, we will start with 157 basic combinations, then multiplied by seven due to Queen substitutions, to obtain 1099 possibilities.
Adding Camel and Giraffe versions of the Princess and Empress has been something of a desperate ploy to increase the number of possible variations, but it has now occurred to me that another new piece, a sort of anti-Griffin, could be added; a piece the move of which is one orthogonal step followed by moving along a diagonal line outwards, at least two steps. A piece of this type has been invented, and given the name of an Aanca; that has been used for something else in a historical chess variant, although that is hardly too much of an objection.
The Camel Princess and the Camel Empress have been used before in chess variants, with the Camel Princess being called the Cavalier and the Camel Empress called the Canvasser.