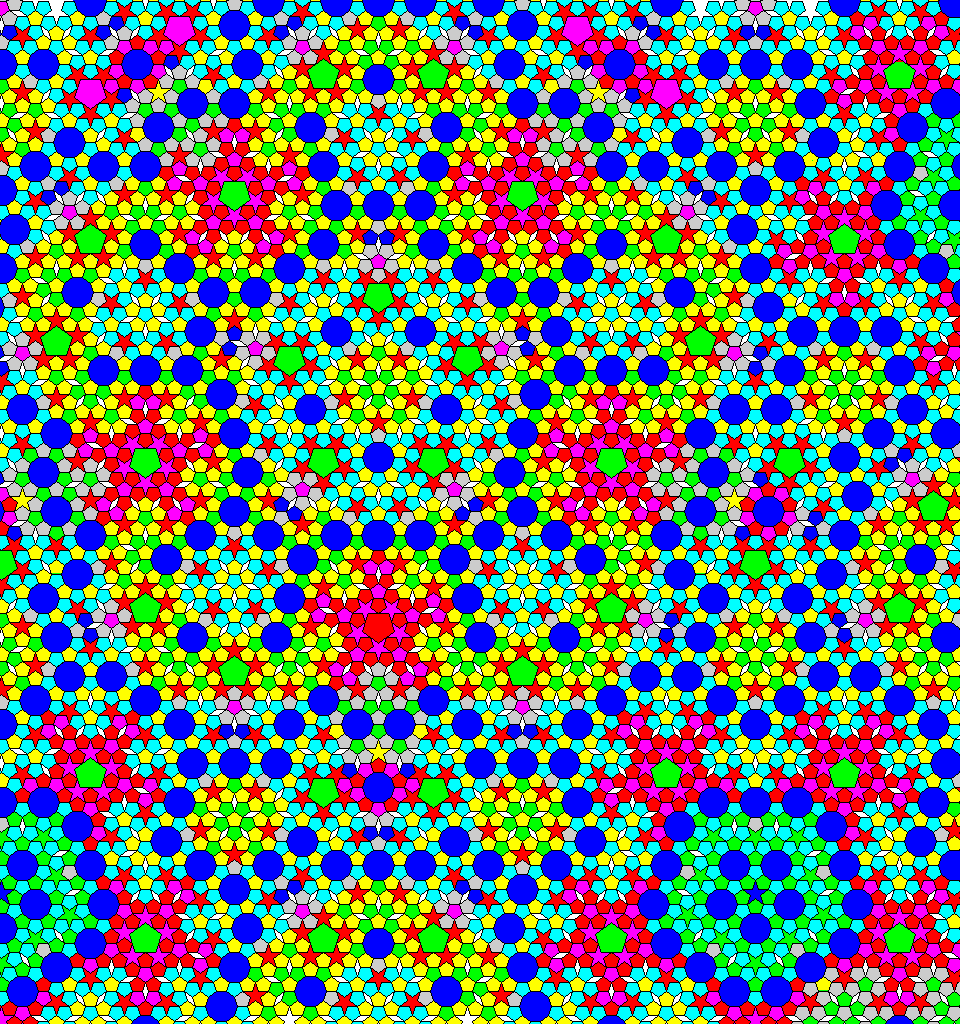
Here is the pentagonal tesselation that appeared on the first page of this section with one difference. Although it seemed the diamond piece cannot be avoided, the "boat", with its lack of symmetry, was a piece I disliked. So, after producing the tesselation, I changed and adjusted some pieces so that every "boat" could be removed, by using decagons, which do have fivefold symmetry, in the tesselation.

Note that while I was able to adjust out the boat pieces in this entire diagram, as this was done on an ad hoc basis, rather than by modifying the pieces used in the underlying recurrence relation, it is not a proof that it is possible to always eliminate the boat piece completely. But such a proof, of course, is provided in the page about Penrose tilings, where the pieces used in the drawing above were used to build up tiles that could be used to tile the entire plane as the components of a Penrose kite and dart tiling.
The starting point for the tiling above is a pentagonal tiling that looks very much like the original Penrose tiling:
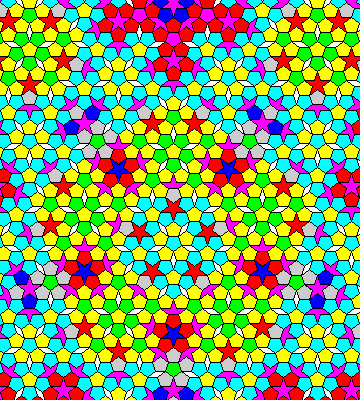
Unlike that tiling, however, the diamonds, when enlarged, are oriented with the star towards the pentagon from which they arose rather than with the boat piece in that direction. Again, this was to delay the use of the less-symmetric boat piece as much as possible. Then, the tiling on the first page was developed by adding double-sized pentagons to the twice-enlarged boat and star pieces, to somewhat reduce the need for boat pieces.
Then, the first step towards modifying that basic tiling was to reduce the number of hat pieces needed by introducing double-sized pentagons, as shown below:
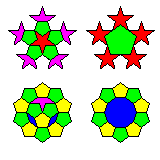
That led to the decorative tiling on the first page, the double-sized pentagons adding to the variety of the pattern. To eliminate the rest of the hat pieces, decagons were introduced, also as shown in the diagram, but although that led to the hat pieces being completely eliminated, it also meant the decagons tended to dominate the arrangement, making it less decorative from my own aesthetic point of view.
This tiling may not have the mathematical elegance of the Penrose tiling, but it certainly has decorative possibilities.

One way to consider the available possibilities would be, by analogy with the hexagon-boat-star tiling for the basic shapes, to consider these shapes, used to supplement a pentagonal tiling, as being associated with a hexagon, a boat, a decagon, and a fat star, as illustrated in the diagram at left.
The boat-only tiling on the previous page, suggestive of fish scales, leads us to ask if we can do without the hexagon, so as to make a tiling from pieces with five-fold symmetry only. Clearly, we would have to exclude the double-sized pentagon to do so; while two adjacent decagons can adjoin one of the points of the fat star, then a hexagon would be needed.
In the case of the star, it is not quite as obvious; five decagons could surround a star. But over a longer range, the tiling would have to be like a tiling with stars and pentagons only, which is not possible.
So, let us perhaps content ourselves with attempting to find a systematic method for tiling the plane with the hexagon, star, decagon, and fat star other than the one already obtained based on the Penrose kite and dart tiling.
Of course, it certainly is possible to tile the plane with pentagons only if the pentagons are not regular pentagons:
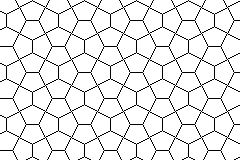
In this well-known example, the symmetry is of a conventional type, with a repeating cell. This particular tiling is sometimes called the "Cairo tiling", because it was noted as being used there in street paving. Trying to begin with three irregular pentagons that begin at a point,

I obtain a tiling with a few hexagons as well. But, trying harder, I finally do attain a tiling with only irregular pentagons and a hexagonal cell; however, no overall reduction in the amount of distortion in the shapes of the pentagons over that in the Cairo tiling is achieved:
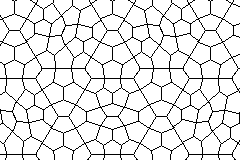
Of course, if one is going to look at conventional tilings, one might as well note the 17 possible symmetry groups available for such tilings,
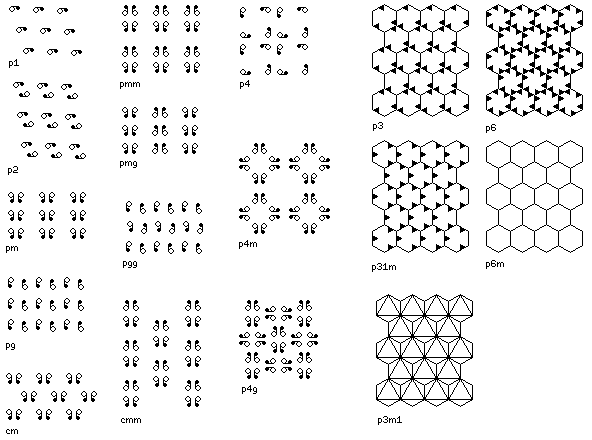
and then, having exhausted the subject (well, not quite, hardly having even scratched the surface of the beauty possible from conventional tilings), hastily depart it.