We have seen the azimuthal projections, which relate the globe to a tangent plane, and we have seen the cylindrical projections, which relate the globe to a tangent cylinder.
A cone is the third developable surface, that is, a surface that can be formed by bending a flat sheet of paper without stretching it, that by virtue of being circular can be placed in contact with an area of interest on the globe.
Since coordinate transformations are usually difficult to carry out (although for some azimuthal projections, there are handy methods of construction available) when projections are used only in their conventional aspect, the azimuthal projections serve for areas around the poles, the cylindrical projections serve for areas near the Equator. Thus, the conic projections serve for everywhere in between, making them very popular in older atlases.
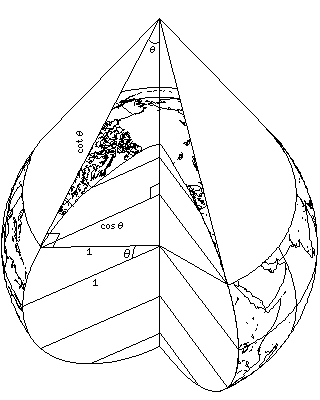
The following diagram

illustrates the principle of conic projections. A cone contacting the Earth at a parallel of latitude, when flattened out, forms a partial circle. The proportion of the circle it makes equals the sine of the latitude of the standard parallel.